This scan provided by Queen's College Library -- thank you!
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Today, let's look at the way that astronomers describe the brightness of a star. It's not quite as straightforward as you might imagine...
Before we get to stars, let's talk about the way people describe the properties of ordinary objects in the everyday world. For example, the mass of different animals.
Suppose we pick three similar animals: a dog, a human, and a cow.
Q: Which animal is the most massive?
Q: Roughly how many times more massive
is a human than a dog?
Note two properties of the "mass" system of measurement:
These seem so obvious that you might wonder why I'm bothering to mention them. Well, as you will see, these properties are NOT shared by the magnitude system.
People in all corners of the world have looked up at the stars (after all, without television, what else could they do at night?). We have detailed records from several cultures in the Middle East; in some, priests spent years studying the motions of the stars and planets, often trying to predict events in the future. Their motives may have been different than ours, but in some cases, they did make very good measurements of what they could see.
Hipparchus of Rhodes compiled a catalog of about 850 stars. He described the brightness of each star by placing it in one of six categories, which one could call "brighest, bright, not so bright, not so faint, faint, faintest." Later scientists used the word "magnitude" to describe these categories.
mag-ni-tude n.
1. a. Greatness of rank or position: "such duties as were expected
of a landowner of his magnitude" (Anthony Powell).
b. Greatness in size or extent: The magnitude of the flood
was impossible to comprehend.
c. Greatness in significance or influence:
was shocked by the magnitude of the crisis.
From the American Heritage Dictionary of the English Language,
Fourth Edition Copyright 2000 by Houghton Mifflin Company.
The brightest stars were assigned to "the first magnitude", just as we would call the best movies or restaurants "first rate." The next-brightest stars were called "second magnitude", and so on down to the faintest stars visible to the unaided eye, which were called "sixth magnitude."
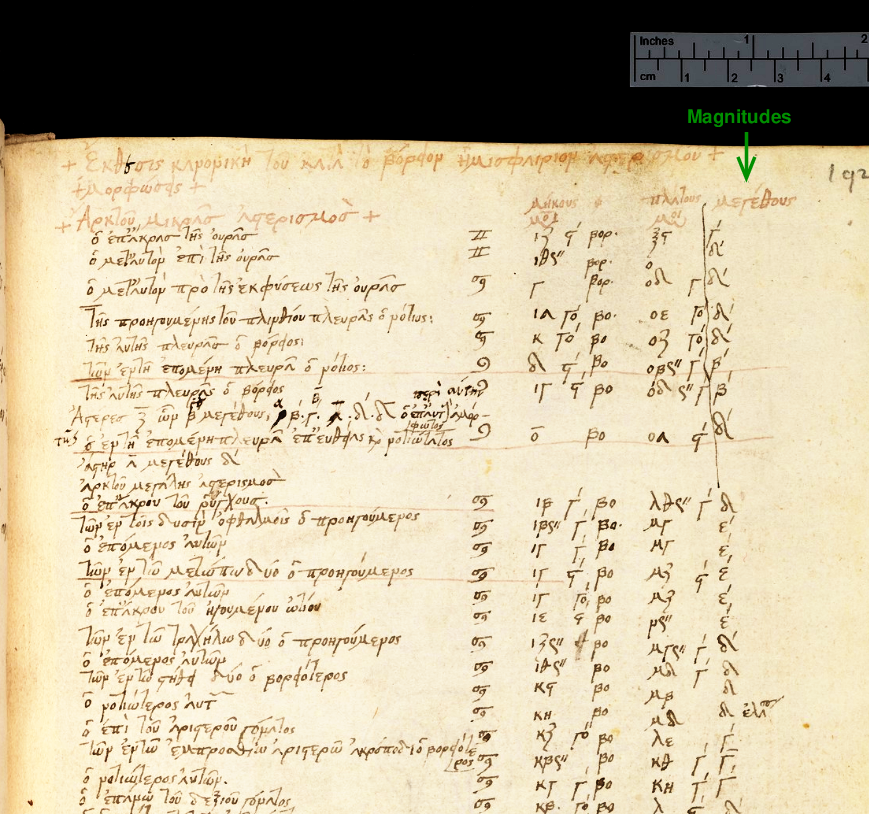
Although no copies of Hipparcos' catalog have survived, his work was incorporated into later Greek writings which we can examine today. Below is a copy of one page from what is one of the greatest astronomical monographs of the ancient world, Ptolemy's Almagest. The rightmost column for each star holds its magnitude.

This scan provided by
Queen's College Library -- thank you!
Q: What are the magnitude values for the first two stars?
Why aren't the values given as numbers?
Well, our modern Arabic numerals hadn't been invented yet. So the Greeks used letters to denote numbers: α = "alpha" for 1, β = "beta" for 2, and so forth. The brightest stars were magnitude 1, and the faintest magnitude 5.
This is the origin of the peculiar convention that
If you want to test yourself sometime, go outside on a clear night and look north. Find the Big Dipper, and use its "Pointers" to locate the Little Dipper.
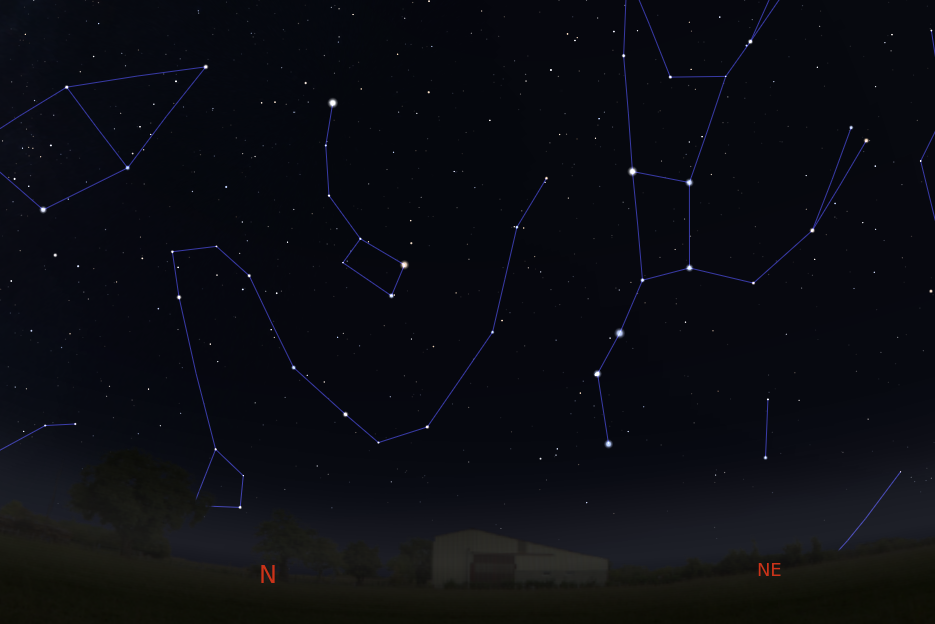
Polaris, the North Star, is the brightest star in the Little Dipper; it shines at magnitude 2 at the tip of the handle. The other stars in the Little Dipper are much fainter -- how faint can you see?
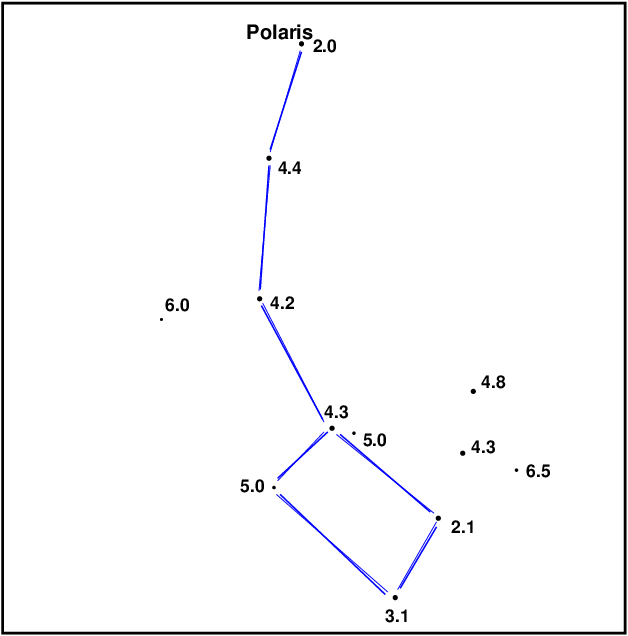
If you see two stars, side by side, and you estimate that the one on the left is about "twice as bright" as the one of the right, does that mean that the star on the left really causes twice as many photons to strike your eye each second? No! Your eyes, like your ears, have evolved to handle a very wide range of environmental stimuli. These human (and animal) senses apparently work on a logarithmic scale.
What's a logarithm? It is a way of re-labelling numbers so that their values are compressed.
original value logarithm base 10
--------------------------------------------
1 0
10 1
100 2
1,000 3
10,000 4
100,000 5
1,000,000 6
--------------------------------------------
Logarithms can save a lot of space and breath. Instead of having to write (or say) 5,248,000,000 = "five billion, two-hundred and forty-eight million," you can simply write (or say) 9.72 = "nine point seven two."
Why do human senses work this way? In the real world, there can be a huge range between, say, the quietest important sound and the loudest important sound.
The sound of the gunshot is about 1014 = 100 trillion times higher in intensity than the sound of a whisper. But humans need to be able to hear both. Audio scientists invented the decibel system to compress this huge range of intensities into a more manageable range. On that scale, a whisper is about 20 decibels, and a gunshot about 160 decibels. Much more convenient ....
If you create two different sounds in a lab, and ask people to turn a knob until one sound is "about twice as loud as the other", you will find that
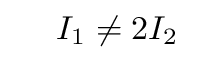
Instead, the relationship will be something closer to
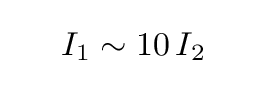
In other words, human perception turns a true ratio of 10 in intensity into a smaller factor. Something similar happens when people try to describe the brightness of lights they see with their eyes.
In the nineteenth century, astronomers devised a number of tools which allowed them for the first time to make accurate, quantitative measurements of stellar brightness. They discovered two properties of the traditional magnitude classifications:
An astronomer named N. R. Pogson came up with a system which would roughly preserve the ancient magnitude values, while allowing modern scientists to extend it to more precise measurements. In a paper published in 1856, he proposed that the magnitude system be defined as follows: given two stars with intensity of brightness I1 and I2, define a magnitude difference which is based on their ratio of intensities:
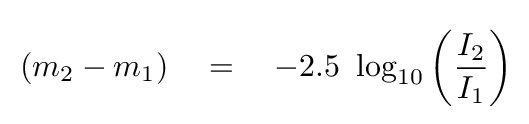
So, for example,
intensity intensity ratio magnitude difference
I(1) I(2) I(1)/I(2) (m1 - m2)
---------------------------------------------------------------
1 0.01 0.01 +5.00
1 0.1 0.1 +2.50
1 0.5 0.5 +0.75
1 1 1 0.0
1 2 2 -0.75
1 10 10 -2.50
1 100 100 -5.00
---------------------------------------------------------------
Q: Star A appears 50 times brighter than star B.
The magnitude of star A is 1.4. What is the
magnitude of star B?
Note again the counterintuitive sign of magnitude differences: the brighter star has a smaller magnitude.
Note also that this definition says nothing about the zero-point of a magnitude: it provides only the DIFFERENCE between two stars. Exactly where to set the zero-point of the magnitude scale is a matter of some debate, and eventually comes down to an arbitrary choice. We'll deal with it later.
If one is given the magnitudes of two stars, one can easily calculate the ratio of their intensities; just invert the above equation to find
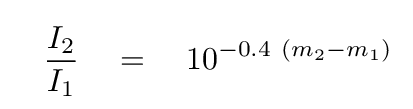
Q: One of the stars in the handle of the Big Dipper is
really a pair of stars, Alcor and Mizar, just far enough
apart for people with good eyes to distinguish.
Given magnitudes of 4.00 for Alcor and 2.06 for Mizar,
how many times brighter is Mizar than Alcor?
Note that even a relatively small difference in magnitudes can mean a very large ratio of brightness. For example, Sirius, the brightest star in the sky, has a magnitude of about -1.4. If you look at a chart of its constellation (Canis Major), it is drawn as the biggest circle:
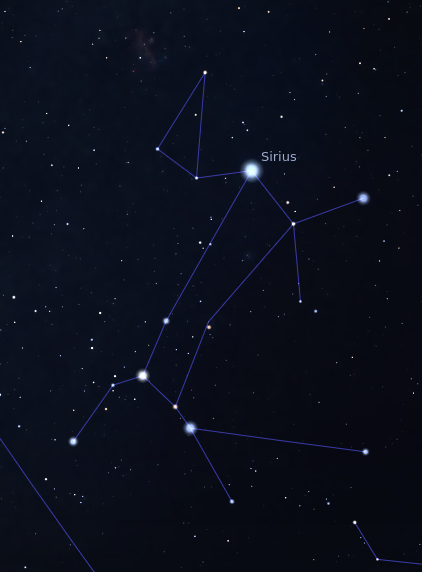
Let's zoom in a bit.
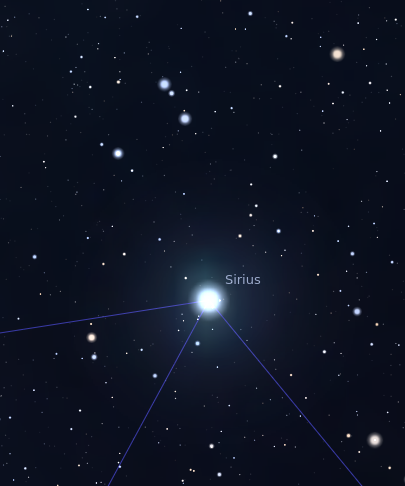
The stars in the little triangle to the upper-left of Sirius are about magnitude 5.
Q: How many times brighter is Sirius (mag -1.4)
than a star of magnitude 5?
Wow! That's much brighter, as you will see at once if you look at a photograph of the area:
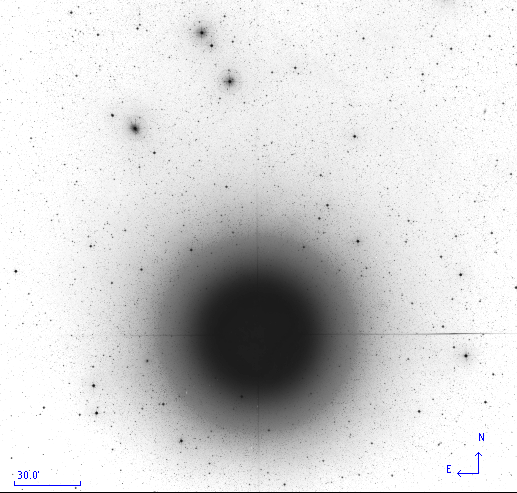
Q: The average diameter of the dark-adapted pupil in a human eye
is about 6 millimeters;
the average person can see a star of magnitude 6 on a clear,
dark night.
If the same person were to look through typical 7x35 binoculars,
how faint a star might she be able to detect?
There are drawbacks to the magnitude system. One of the big ones is the work one must do when trying to figure out the result of adding or subtracting two stellar sources, rather than multiplying or dividing them. Suppose there are two stars, A and B, with magnitudes m(A) and m(B), which appear so close together that their light blends into a single source. What is the magnitude of the resulting blend?
m(A + B) =? m(A) + m(B) NO!
The proper way to do this calculation is to convert the magnitudes back into intensities, add together the intensities, and then convert back into magnitudes. There's no way around it.
Q: My eyesight is so poor that I can't distinguish Alcor from
Mizar without my eyeglasses.
Given magnitudes of 4.00 for Alcor and 2.06 for Mizar,
what is the magnitude of the single blurry object I see?
Why do we continue to use this system? There are several reasons:
Astronomers who study objects outside the optical wavelengths -- in the radio, ultraviolet, or X-ray regimes -- do not have any historical measurements to incorporate into their work: these fields are all very recent, dating to the 1930s or later. In those regimes, measurements are almost always quoted in "more rational" systems: units which are linear with intensity (rather than logarithmic) and which become larger for brighter objects. In the radio, for example, sources are typically measured in janskys, where
1 Jansky = 10^(-26) watts / square meter / Hertz
A source of strength 5 Janskys is 5 times brighter than a source of 1 Jansky, just as one would expect.
Take a look at this photograph of open star cluster M103.
Which are the three brightest stars? Clearly, these stars should have the smallest magnitudes, right? And here are the Bt magnitudes in the Tycho-2 catalog.
star Bt mag --------------- A 7.6 B 8.6 C 9.3 D 12.6
But watch what happens when we take a picture of the same cluster of stars with a different sort of photographic emulsion. (Click on the picture below)
Which are the three brightest now? So which should have the smallest magnitude values?
Okay, something's fishy here: how can one set of stars be brightest in one picture, but a different set of stars be brightest in another?
Stars come in a wide variety of colors. It's not glaringly obvious when you look at them up the in sky, for several reasons:
Nonetheless, if you look carefully, you will see slight differences in colors. In the constellation Orion, for example,


Images courtesy of Denys Sakva
Betelgeuse, at the upper left, is clearly redder than most of the stars.
You can enhance the colors in a photograph (or an eyepiece) by defocusing so that the light is spread out over a larger area.

Image from
Astronomy Picture of the Day 2004 July 8 , copyright
Stefan Seip .
Q: What causes some stars to look blue and others red?
In other words, what is the cause of stellar color?
The answer is temperature.
Let's take just a moment to discuss the way astronomers describe temperature. Here on Earth, under ordinary circumstances, people use temperature scales which tend to give "convenient" values for most objects: small positive numbers, like "12" or "45" or "98".
Fahrenheit Celsius
-----------------------------------------------
temp = 0 salty water pure water
freezes freezes
temp = 100 human body pure water
temperature boils
------------------------------------------------
Astronomers study objects which cover a much, much wider range of temperatures. Very cool clouds of gas and dust may have temperatures which are inconveniently low in this ordinary scales: -420 Fahrenheit or -250 Celsius. Ordinary stars like the Sun have temperatures in the thousands of degrees, and very hot stars may reach many tens of thousands. It is confusing to deal with both negative and positive numbers, so astronomers choose to adopt the Kelvin temperature scale: it starts at the coldest possible temperature, then moves upwards in steps which are the same size as Celsius degrees.
Fahrenheit Celsius Kelvin
-----------------------------------------------------------
coldest possible -459 -273 0
temperature
pure water
freezes 32 0 273
pure water
boils 212 100 373
------------------------------------------------------------
It's easy to convert from Celsius to Kelvin: just add 273. Converting from Fahrenheit to Kelvin is a bit harder, but if you use Celsius as an intermediate step, it isn't so hard.
Note that scientists often omit the word "degrees" when describing a temperature in Kelvin. Pure water freezes at "0 degrees Celsius," but at "273 Kelvin". Someone who wants to sound old-fashioned might even write "273 Kelvins".
You probably already know that hot objects glow with a color that depends on their temperature. Relatively cool lava -- only 1000 Kelvin -- appears a dull red:

while an oxyacetylene blowtorch at 3200 Kelvin emits bluish-white light:

What's happening is that the temperature of an object affects the spectrum of light that it emits. A "spectrum" is simply a description of the amount of light broken up by wavelength. We can display the spectrum of an object as a picture, like this:

Image copyright Maurice Gavin
We can also show spectra in graphical format, with wavelength along the horizontal axis and intensity of light along the vertical axis.

Image and spectrum copyright Maurice Gavin
It turns out that there is a clear pattern to the spectrum of light emitted by an object.
The formal definition of magnitudes describes only differences between two stars; it does not contain a zero point. That means that we can only write statements such as
Sirius is 0.30 magnitudes brighter than Canopus
It would be much more convenient if we could assign an unambiguous number to each star individually, like this:
Sirius has magnitude -1.46
In order to turn all our relative measurements into fixed magnitudes, we need to choose one zero point for the magnitude scale. But how?
One of the complicating factors is that the apparent brightness of a star depends on the equipment being used to measure it. "Sure," you might say, "when I look through a big telescope, a star will appear much brighter than when I look through a small telescope." True enough. But the relative brightness of two stars right next to each other may change, depending on how one views them:
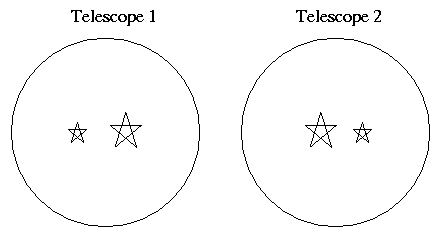
Why?
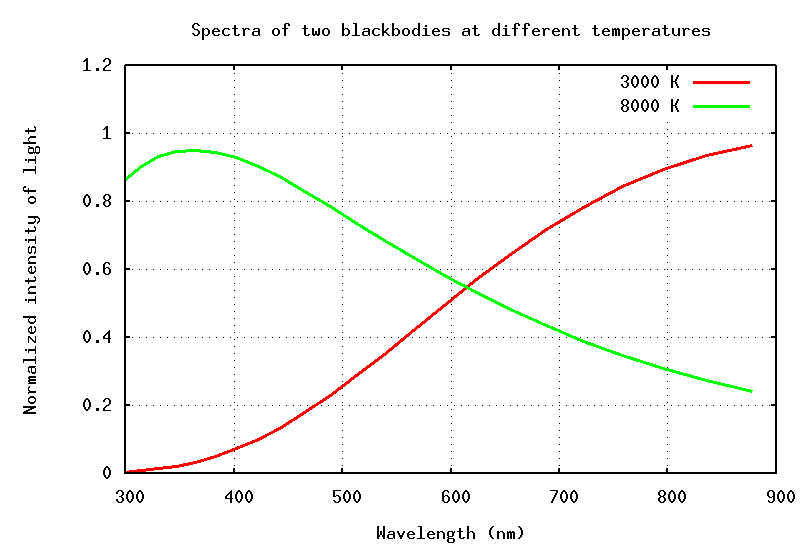
The answer is that the temperatures of stars span a wide range, from less than 2000 Kelvin to more than 50,000 Kelvin. Most stars emit radiation like a classical blackbody, with a spectrum which depends mostly on their temperature:
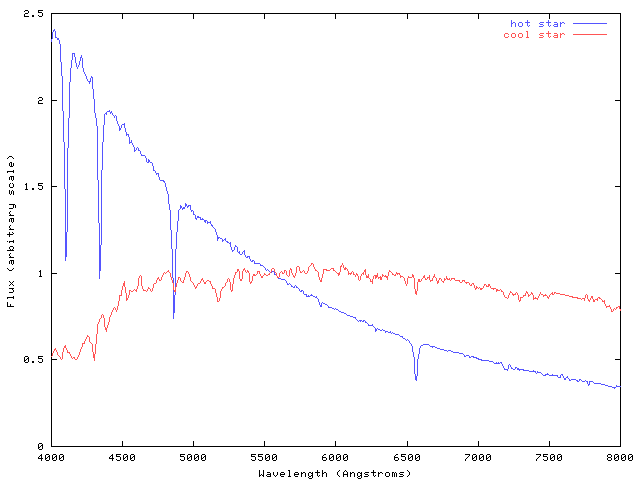
Now, if one were to attach a blue filter to one's telescope, one would measure only the blue light emitted by stars. In that case, the hot star would appear brighter than the cool one:
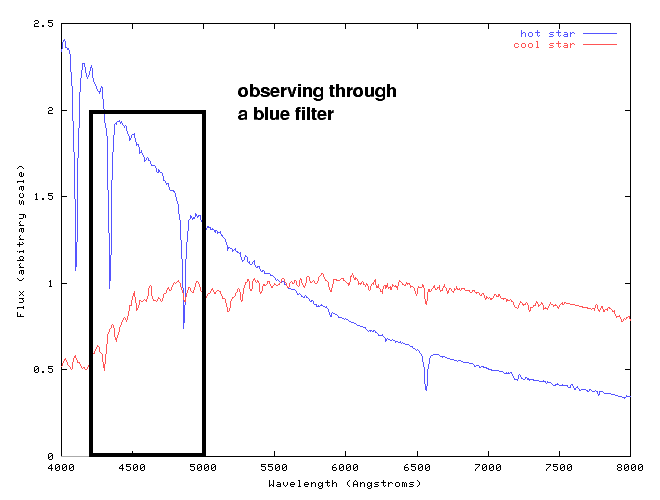
On the other hand, if one were to attach a red filter to one's telescope, the cool star would appear brighter than the hot one:
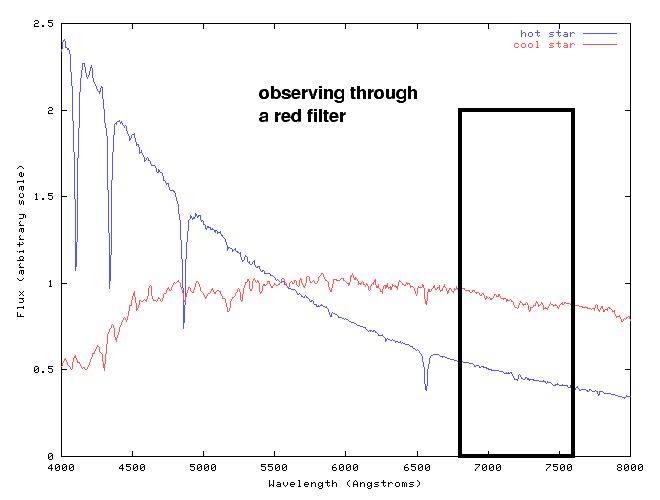
So, the ratio of apparent brightness -- and, hence, the magnitude difference -- between two stars depends on the bandpass through which one observes them. A "bandpass" is the overall sensitivity of an instrument as a function of wavelength: it includes the effects of filters, plus characteristics of the detector, and telescope mirrors.
Astronomers have settled on a number of different photometric systems, each one based on a particular passband (i.e. a particular combination of filter and detector and telescope). One should always remember to specify the system when quoting the magnitude of a star.
Most astronomers working in the optical use the UBVRI photometric systems. These are five different passbands which stretch from the blue end of the visible spectrum to beyond the red end. They were set up many years ago by several astronomers:
The systems are defined by particular combinations of glass filters and photomultiplier tubes, since there were created back in the days before CCDs existed. Since photomultipliers and CCDs have very different spectral sensitivities (photomultipliers are more efficient in the blue, CCDs in the red), it is difficult to make the effective passband of a CCD-based instrument match that of a photomultiplier-based instrument. In 1990, Michael Bessell came up with a recipe for making filters out of common colored glasses which would reproduce pretty closely the official Johnson-Cousins UBVRI passbands.
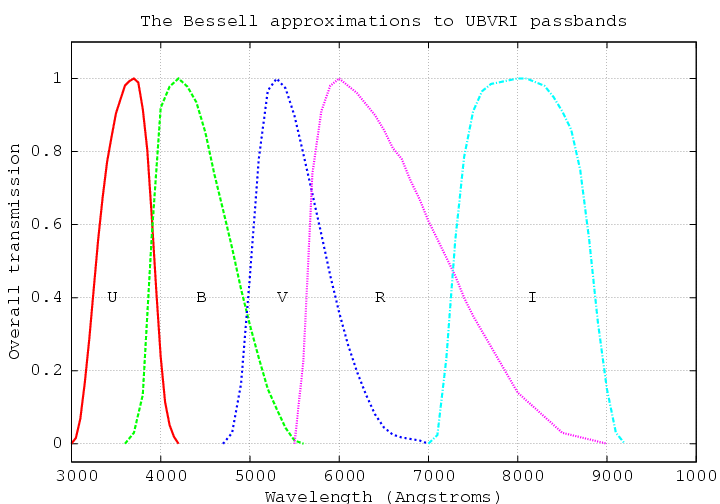
The UBVRI passbands are called broadband because they span wide swaths of wavelengths. The spectral resolution of the passbands is small:
central wavelength
spectral resolution = ---------------------- = approx 5
width of passband
For some applications, astronomers use filters which transmit a much smaller range of wavelengths; a common filter used to measure light emitted by hydrogen atoms is centered at 6563 Angstroms and roughly 20 Angstroms wide:
6563 Angstroms
spectral resolution = ---------------------- = approx 330
20 Angstroms
A narrowband filter like this requires much longer exposure times to build up the same signal as a broadband filter. Since telescope time is so precious, astronomers tend to use broadband systems. That's one reason for the popularity of the UBVRI system.
When writing the magnitude of a star, astronomers use an abbreviation to denote the photometric system of the measurement:
V = 1.03 means "magnitude of this star in the V system is 1.03"
B = 0.46 means "magnitude of this star in the B system is 0.46"
There is also a convention to use lower-case letters for raw measurements and upper-case letters for fully reduced values:
b = 1.18 means "a measurement made through a B filter"
B = 1.22 means "the same measurement after a full reduction"
So, once one has settled on the equipment one will use -- which sets the photometric system -- one still faces the question of the magnitude zero-point. The choice is arbitrary. Astronomers have chosen to use the bright star Vega as their starting point.

In the UBVRI systems, the star Vega is defined to have a magnitude of zero.(*)
* Actually, this is not quite true: the zero point is defined strictly by the mean measurements of a set of bright stars (which may include Vega), rather than by Vega alone. However, since Vega always ends up with a magnitude within a few percent of zero, the simple rule "Vega's magnitude is zero" suffices for almost all purposes.That is,
Vega's magnitude in U-band: U = 0.0
Vega's magnitude in B-band: B = 0.0
Vega's magnitude in V-band: R = 0.0
Vega's magnitude in R-band: V = 0.0
Vega's magnitude in I-band: I = 0.0
When ordinary people use the word "color" to describe a star, then mean "what is the tint perceived by the eye?" One star might have a color of "pale orange", another "bluish-white".
But astronomers use the word "color" in very different way. To them, "color" is a measure of the magnitude difference of a star in two passbands .... relative to the magnitude difference of Vega in the same passbands. Let me illustrate with an example or two.
Consider the stars Vega (a hot star), Antares (a very cool star), and Alnitak (a very hot star in the constellation Orion).


Image of Vega from
Scott Atwood
Image of Scorpius from
Friends of Rondeau
Image of Orion from
starrynightphotos.com
We can measure their magnitudes in the B and V passbands.
B V (B-V)
-----------------------------------------------------------
Vega 0.00 0.00 0.00
Antares 2.96 1.09 +1.87
Alnitak 1.59 1.79 -0.20
An astronomer would say, "Antares has a color index of (B-V) = 1.87", or less formally "Antares has a color of 1.87", or, even less formally, "Antares is red." Any color index less than about 0.5 (indicating a temperature similar to Vega's roughly 10,000 K) means a star is "blue"; any color index greater than about 1.5 (indicating a temperature much less than Vega's) means a star is "red".
Because so many measurements have been made in the B and V systems over the years, astronomers often use the (B-V) color index as "the" measure of the color of a star. In fact, one can also refer to the color index formed by (U-B), or (V-I), or any other pair of passbands. But (B-V) is the most commonly used index. For example, the Hertzsprung Russell diagram, which shows the relationship between the luminosity of a star and its temperature, is usually plotted with color index (B-V) as an indicator of temperature:

Image courtesy of
Richard Powell and "An Atlas of the Universe"
Stars on the main sequence typically emit light like blackbodies, so there is a pretty simple relationship between color index and temperature. The figure below, based on data compiled by Pecaut and Mamajek, ApJS 208, 9 (2013) , allows one to convert quickly from color index to temperature or vice versa.
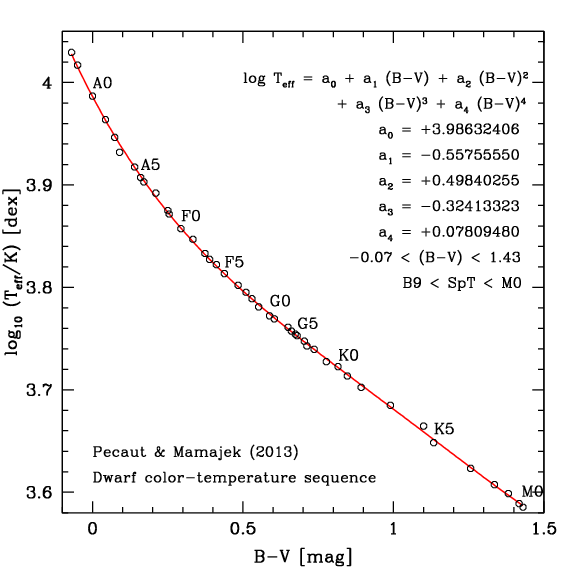
Image courtesy of
Eric Mamajek
The Sun has a spectral type of G2.
Q: What is the (B-V) color of the Sun?
Q: What is the temperature of the Sun?
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.