 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Investigating planets around other stars
Look at the measurements of a star called "tau Bootes".
This star moves in a circle with a period of P = 3.312 days
and a speed of about v = 466 m/s.
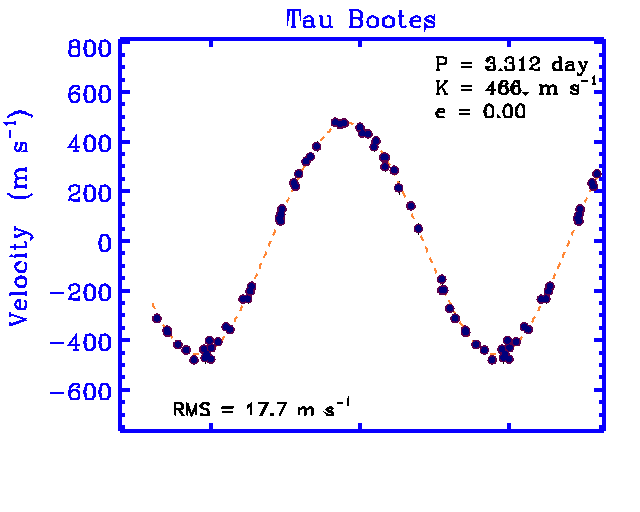
- What is the circumference of the star's orbit?
In other words, how far does the star travel
as it makes one complete circle?
- What is the radius Rs
of the star's orbit around the center of mass?
- The planet is circling the star with a much larger
orbital radius Rp, but
with exactly the same period P.
Use Kepler's Third Law to figure
out the radius of the planet's orbit.
You can assume that
- (Rp + Rs) is roughly (Rp)
- the mass of the star is about the same as the mass of the Sun
- How does the planet's orbit compare in size of the
orbit of the Earth around the Sun?
- Use the ratio of orbital radii to figure out
the ratio of masses.
Then estimate the mass of the planet.
- How does the planet's mass compare to the mass of the Earth?
- Would you like to live on this planet?
For more information
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.

 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.

 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.