Tools: Geometry and Time

Geometry

Astronomers, like most other scientists, describe the world in the language of mathematics. You won't see a great deal of math in this course ...

if you DO like math, consider switching to SPSP 301, University Astronomy; it meets MTWR 5:00 in 08-A300

... but one bit of Euclid's lingo which you must be able to understand is a simple bit of geometry. Namely, the technique by which one can figure out the length of one side of a right triangle. Astronomers use this technique to determine distances to celestial objects -- by measuring their parallax.

• Read this description of using parallax to measure distances

In order to use this technique to calculate a distance, you need to know (at least) two things:

1. a baseline distance
2. an angle

So, the basic idea is to look very carefully at the same object from two different places -- that gives you the baseline. If you can detect a shift in the object's position relative to the background, you have the angle. And then you can find the distance.

You will have a chance to use the "parallax" technique to measure a distance in class today. Not the distance to Alpha Centauri, or Saturn, or the Moon; no, you will attempt to measure the distance to your thumb.

Measuring the distance to asteroid 2002 NY40 via parallax

In the early morning hours of August 16/17, 2002, I participated in an experiment to measure the distance to asteroid 2002 NY40, which was zipping past the Earth at a relatively small distance. I took pictures of the asteroid from the RIT Observatory, in Rochester, NY. At the very same time, astronomers at the US Naval Academy in Annapolis, MD, were also observing 2002 NY40. You can read a full description of our measurements, but the essentials are contained in the pair of images below (click on the picture).

The asteroid (which you can recognize by its slight trail) appears to move by about 40 arcseconds from one image to the other. That's a very small shift -- only about 0.011 degrees. Can you estimate the distance to asteroid 2002 NY40, based on this information?

Time

Time is pretty important to astronomers. Why? Two reasons:

1. Some objects change their positions or brightnesses over time. Planets, for example, orbit around the sun with periods of months to years. Some stars pulse, expanding and contracting, brightening and dimming, very regularly with timescales of days to weeks.

2. The Earth rotates on its axis, causing objects to rise above the eastern horizon, wheel across the sky, and set below the western horizon. If you want to study some particular star, you need to know not only where to look for it in the sky, but when as well.

Astronomers have always been good time-keepers. In fact, during the fifteenth through nineteenth centuries, they were given the very important job of keeping the official time in most European (and American) countries. Back then, ships needed to re-set their clocks every time they came into port. Navigators needed to know the time to figure out their longitude. Without an accurate time, captains didn't know exactly where they were ... which could lead to disaster.

But how many different kinds of time are there?

Solar time

Keep track of the intervals at which the sun passes highest in the sky, around noon.

Sidereal time

Keep track of the intervals at which a star passes highest in the sky.

Atomic time

Keep track of the vibrations of a collection of atoms under very stable conditions.

• A history of time keeping, from NIST
• Research into "Cesium Fountain" clocks at the USNO

Using time to measure the size of an asteroid

One example of the use of time in astronomy occurred just a few nights ago. Just after midnight on Friday, September 6 (so, really, Saturday, September 7), Prof. Tracy Davis and I watched as asteroid 10 Hygeia passed in front of a star.

Scientists used the orbit of the asteroid to predict that it would block the light of the star TYC 1244-00565-1 around midnight (EDT). They predicted that the shadow of the asteroid would move up the eastern coast of the US at a speed of about 2.96 km per second.

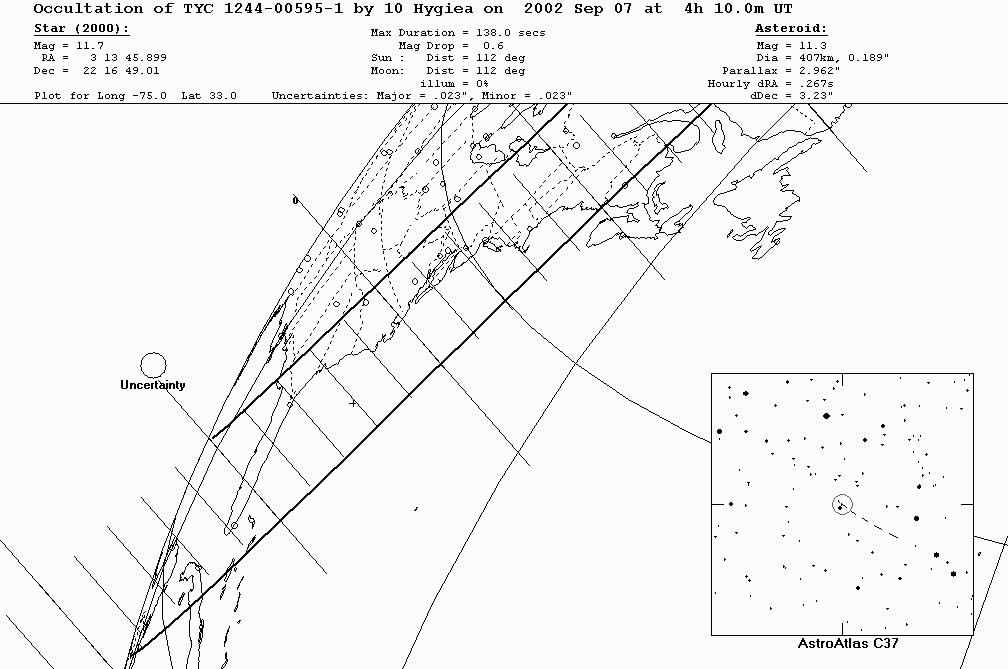
We used the RIT Observatory's 10-inch telescope

and CCD camera to take pictures of a very small area around the asteroid. The exposure time was just one second, and we were able to acquire one image every 5 seconds. Here's an example:

We took a series of about 200 images, starting at 11:52:48 PM. Astronomers had predicted that when the asteroid passed exactly in front of the star and blocked its light, the brightness of the star+asteroid would drop by about 50 percent. If you click on the image above, you'll see a short movie made from a sequence of our images near the predicted time of the event. Can you detect any changes in the brightness of the lower object?

It's easier to detect if you look at a graph of brightness versus time:

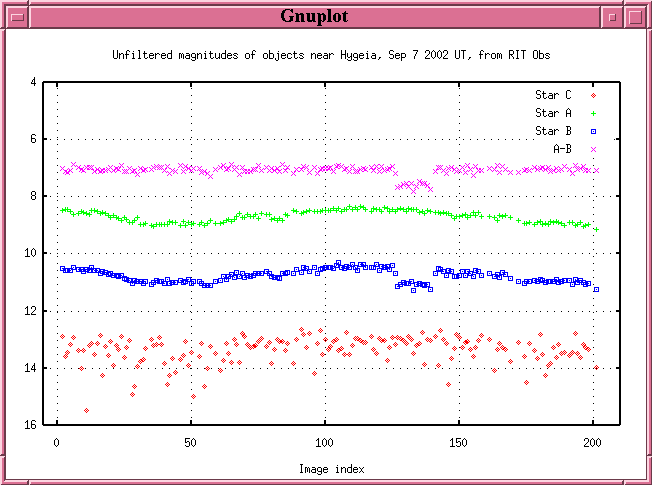
The graph below shows a closeup of the measurements made during the occultation. How large was the portion of asteroid Hygeia which passed over Rochester?

For more information

Copyright © Michael Richmond. This work is licensed under a Creative Commons License.