Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
First, we need to go over the structure of this course.
The "big" topics this course will cover are
There will be some math. It's hard to talk about astronomical objects without using numbers, and whenever numbers are involved, there will be calculations. Most of our work will be limited to arithmetic (+, -, *, /, square roots, etc.) and trigonometry (sines, cosines, hypotenuses, etc.). You will need a calculator. Bring your calculator, paper, and pen/pencil to class.
If you find that there is not enough math for you, perhaps you might consider switching to PHYS-220, "University Astronomy." That course is designed for science majors, and is the first course one would take if starting the Astronomy Minor at RIT. If you want to know more, talk to me outside of class.
Space is big. Really big. You just won't believe how vastly, hugely, mind-bogglingly big it is. I mean, you may think it's a long way down the road to the chemist, but that's just peanuts to space.
Douglas Adams, "Hitchhiker's Guide to the Galaxy
How vast those Orbs must be, and how inconsiderable this Earth, the Theatre upon which all our mighty Designs, all our Navigations, and all our Wars are transacted, is when compared to them. A very fit consideration, and matter of Reflection, for those Kings and Princes who sacrifice the Lives of so many People, only to flatter their Ambition in being Masters of some pitiful corner of this small Spot.
Christiaan Huyghens, Cosmothereoros (1698)
Let's begin by considering the size of space -- the sheer immensity of the universe in which stars, galaxies, and planets exist. As you will see, we will need to adopt some new units to make our task of describing the solar system easier to handle.
We'll start with the Earth. Good ol' Earth. You know this planet well -- you've been living on it for your entire life. How big is the Earth?
I have here at the front of the room a globe: a smaller version of our Earth. The radius of the globe is quite a bit smaller than that of the Earth: it's only about 13 cm.
I also have a little model of the Moon, a ball about one-quarter the size of the Earth.
Q: How far from the globe should I place the ball
in order to show the proper scale of the distance
between the Earth and the Moon?
This isn't far enough. We need to zoom out.
Ah, there's the Moon!
The Moon orbits the Earth at a distance of about 60 Earth radii. Since our little model of the Earth is about 0.13 meters in radius, that means we should place the little ball representing the Moon at a distance of about 7.8 meters from the globe.
Q: How far from the globe should I place the ball
in order to show the proper scale of the distance
between the Earth and the Moon?
Not much! A whole lot of empty space.
But we're just getting started!
You may have heard that the planet Mars recently came especially close to the Earth. It's true: although Earth catches up to Mars and passes it in its orbit once every 780 days or so, not all those passes are the same. The orbit of Mars has a rather high eccentricity -- it's not a perfect circle, but a rather squished ellipse. That means that it sometimes comes closer to the Sun (and the Earth), and sometimes runs farther away. This summer, Mars just happened to be almost as close to the Sun (and the Earth) as it ever gets, just as the Earth passed it.
That means that Mars appeared to grow larger than usual as seen through a telescope.
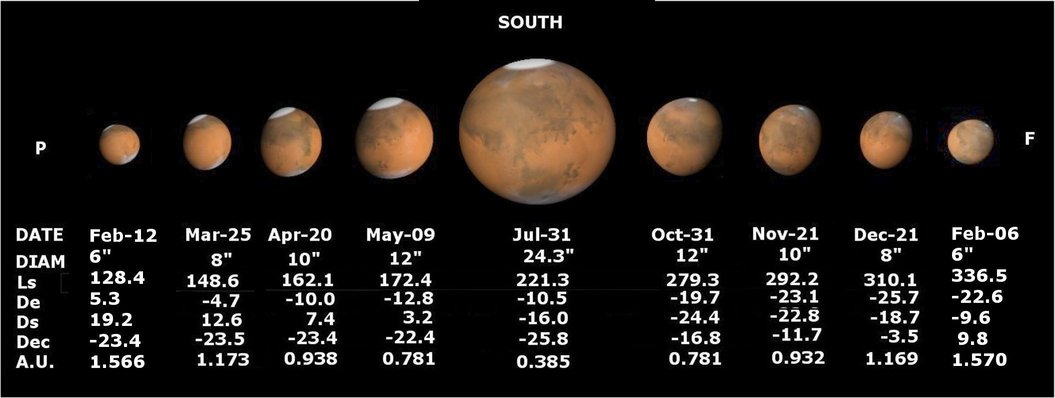
Image courtesy of
Association of Lunar and Planetary Observers
Mars is somewhat smaller than the Earth, so I can represent it with a ball about 7 cm in radius.
Q: How far from the globe should I place the ball
in order to show the proper scale of the distance
between the Earth and Mars, during this close approach?
The correct answer is ... far, far away. At closest approach, Mars was about 57.6 million km from the Earth, so using our scale model,
6 1 Earth radius 0.13 m
distance to ball = 57.6 x 10 km * --------------- * -------------
6378 km 1 Earth radius
= 1200 m = 1.2 km
What about some other bodies in our solar system? The Sun, for instance. You know the Sun is much larger than the Earth, right? Relative to the globe, a big glowing sphere to represent the Sun would have to be about 14 meters in radius.
Q: How far from the globe should I place the giant
glowing 14-meter sphere in order to show the proper
scale of the distance between the Earth and the Sun?
The answer is ... near the Marketplace Mall.
Now, in real life, the Earth-Sun distance is about 149,600,000 kilometers, or 149,600,000,000 meters. That's a lot of digits to write, and read, and remember. It's pretty clear that neither the meter nor the kilometer is a good choice for a unit to describe the distances between objects in our solar system. So, many years ago, astronomers created a unit which is designed specifically for this purpose:
The Astronomical Unit (AU) is defined as the semi-major axis
of the Earth's orbit around the Sun; in other words, roughly the
average distance between Earth and Sun.
1 AU = approx 149.6 million km
If you want to know the exact value, take a look at a table of constants from JPL's Horizons ephemeris system.
Using this special unit, we can describe distances using numbers that range between 0.1 and 100 -- easy to read, and write, and say.
Mars' closest approach in 2018 0.39 AU
Earth-Sun distance 1.00 AU
See? Easy-peasy.
But the solar system extends far beyond Mars, of course. Consider the icy-cold world we call Pluto:
Image of Pluto courtesy of
NASA/Johns Hopkins University Applied Physics Laboratory/Southwest Research Institute/Alex Parker
Let's continue to use our little globe as a scale model of the Earth. On this scale, Pluto would be a little sphere about 2.4 cm in radius, just a bit bigger than a ping-pong ball.
Q: How far from the globe should I place a little
ball in order to show the proper scale of the distance
between the Earth and Pluto?
(If you have a calculator, and you want to do a bit of work,
the real distance is about 5.98 billion km)
Nope. Pluto orbits the Sun at about 5.98 billion km = approx 40 AU, which means that in our model, the little ball sits out in Syracuse.
9 1 Earth radius 0.13 m
distance to Pluto = 5.98 x 10 km * --------------- * -------------
ball 6378 km 1 Earth radius
= 122,000 m = 122 km
I hope you are starting to appreciate the enormous size of space.
But -- hey! So far, we've only considered objects in our own solar system, orbiting our own Sun. There are lot of other stars out there, too. We won't talk much about them in this course; you'll have to wait for the spring semester's PHYS 104, "Introduction to Stellar Astronomy." But let's make a brief detour, just for a few minutes.
The Alpha Centauri star system, which contains three stars, is the closest to our Sun. We'll talk more about this system when we discuss the planet circling one of its components, Proxima Centauri b, later in the course.
Image credit:
ESO/M. Kornmesser
How far is this CLOSEST star system from the Earth? Let's continue to use our globe to set the scale.
Q: How far from the globe should I place a 14-meter
sphere in order to show the proper scale of the distance
between the Earth and Alpha Centauri A?
On this scale, the nearest star system would be more than twice as far away as the Moon! The real distance to this star is so large that plain old meters or kilometers just won't cut it:
Distance to Alpha Centauri system = 41,300,000,000,000,000 meters
41,300,000,000,000 kilometers
Even using Astronomical Units doesn't help all that much:
1 AU
Distance to Alpha Centauri = 41,300,000,000,000 km * -----------------
149,000,000 km
= 276,000 AU
We need a new unit to make inter-stellar distances easy to read and write. Astronomers have devised two, and you may see these occasionally during this course.
The light year (ly) is the distance light travels in one year.
1 ly = approx 9,443,000,000,000,000 meters
The parsec (pc) is the distance at which a distance of 1 AU
subtends an angle of 1 arcsecond.
Read this for an explanation
1 pc = approx 30,860,000,000,000,000 meters
If we use these units, then the distance to Alpha Centauri is nice and easy to write:
1 ly
Distance to Alpha Centauri = 41,300,000,000,000 km * ---------------------
9,443,000,000,000 km
= 4.4 ly
Q: What is the distance to Alpha Centauri in parsecs?
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.