 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
So, suppose that we point our telescopes towards some distant star. It is so bright, and any possible planets around it are so faint, that we can only detect the light of the star itself.
Rats.
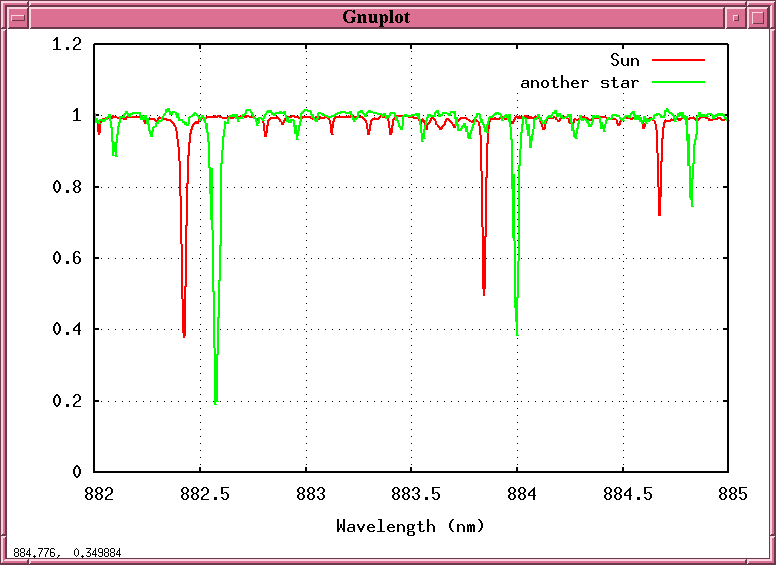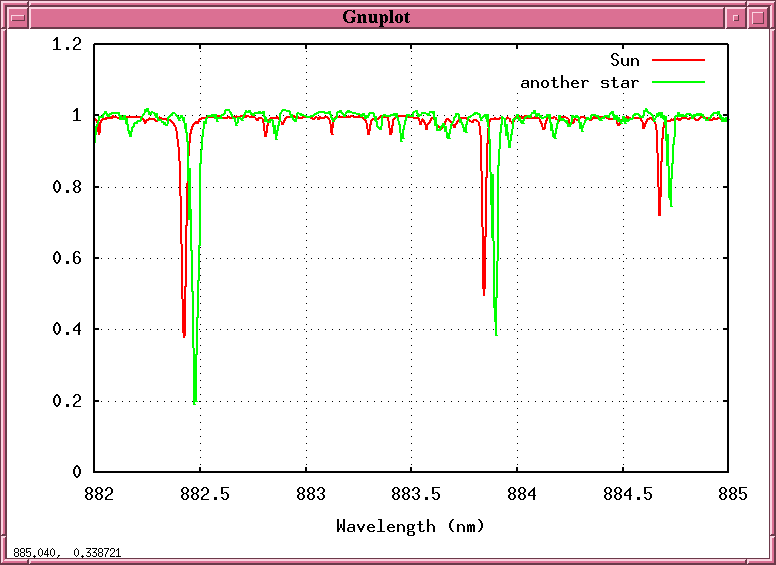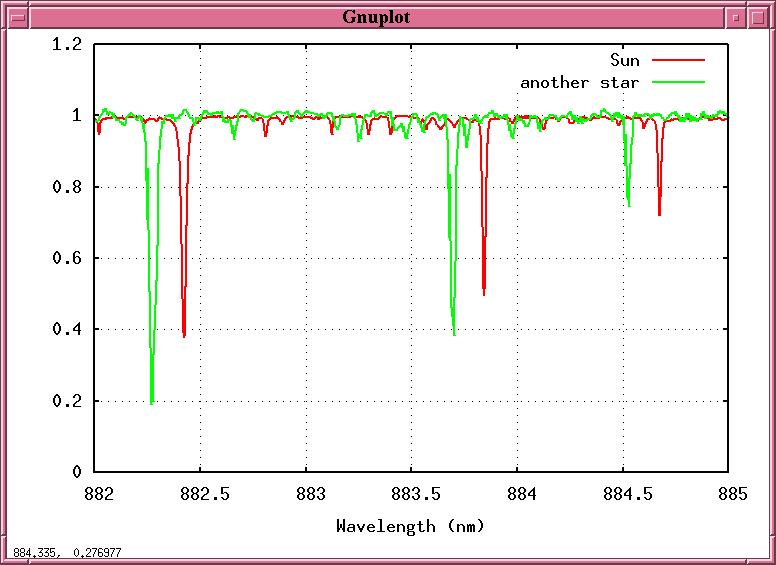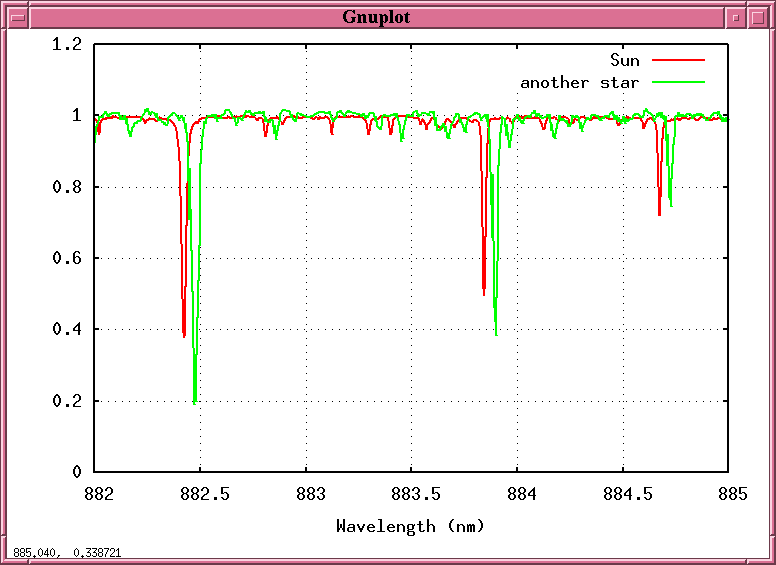
But suppose that we split this starlight into a spectrum and record it -- not just once, but over and over again, night after night, week after week. Maybe, just maybe, we might see the spectrum of that starlight change ....

Q: Why should the starlight shift back and forth,
back and forth, in a periodic fashion?
A: Because the star is moving towards us and
away from us, towards us and away from us,
as it circles around the center of mass
between it and a planet!
A little animation illustrating radial velocity curves
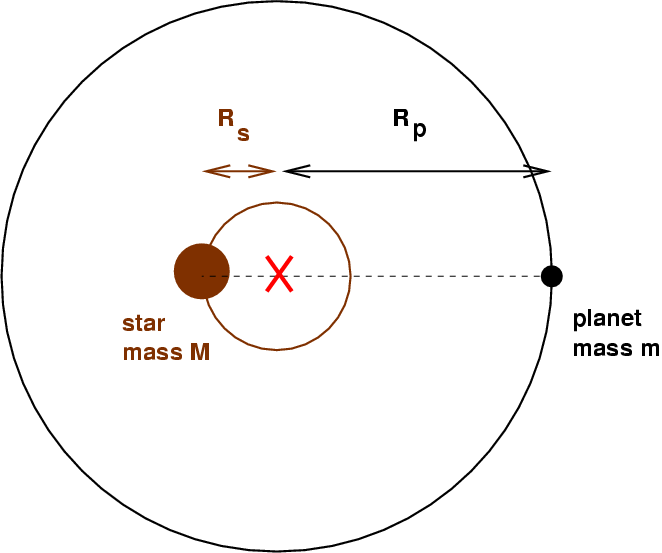
If you could figure out the size of the star's orbit, Rs, and if you knew the size of the planet's orbit Rp -- which is always much, much, larger -- then you could figure out the ratio of orbital radii, and, thanks to center of mass, the ratio of masses.
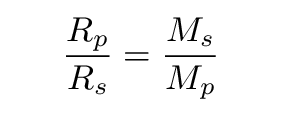
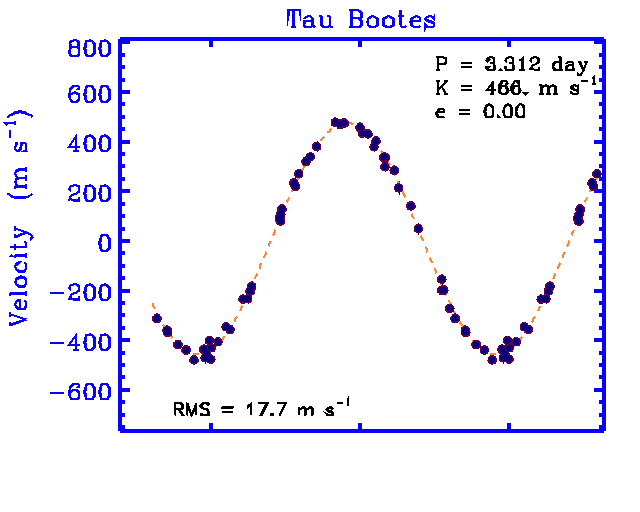
Look at the measurements of a star called "tau Bootes". This star moves in a circle with a period of P = 3.312 days and a speed of about v = 466 m/s.
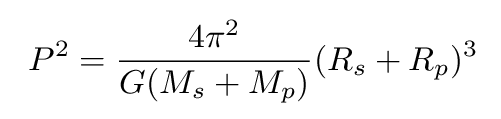
You can assume that
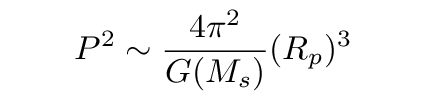
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.