Status report for Sep 09, 2003

The testbed

The idea is to place stars of a range of spectral types in every filter and chip. Specifically,

• one star at the center of every optical filter
• 4 stars spaced equally across every near-IR filter

I have created an artificial catalog of stars near (RA = 0, Dec = 0) which fall on this quadrant:

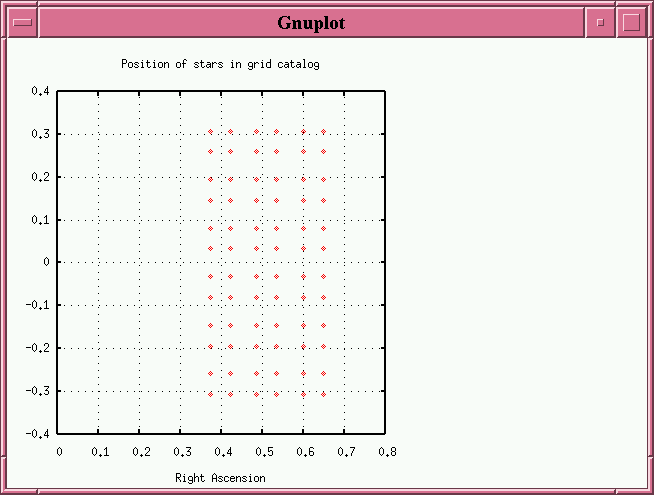
The catalog is very artificial: dwarf stars of spectral type O5, B5, A0, A5, F0, F5, G0, G5, K0, K5, M0, M5 all sit at the same RA and Dec, on top of each other. For the current testing purposes, this is fine; but when the simulator is improved to take realistic "CCD images", the stars will have to be moved so that they are some distance away from each other.

What is the effect of angle-of-incidence on the photometry?

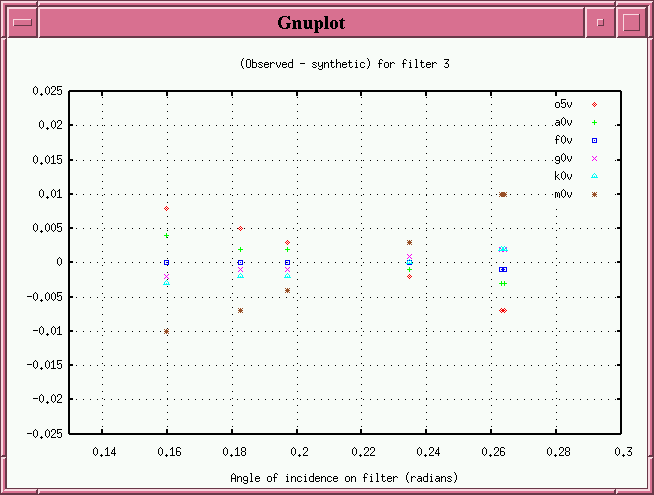
The filter passbands are shifted slightly if light enters the filter at an angle. How large is this effect, and how does it depend on spectral type? I assumed that all filters in a given passband were manufactured to be identical, and designed to have the fiducial transmission curves near the middle of the annulus. I computed the following quantities:

• "synthetic magnitude": convolve spectrum with fiducial filter passband (no shift due to angle of incidence)
• "observed magnitude": convolve spectrum with effective filter passband, shifted due to angle of incidence
• delta = (observed - synthetic) magnitude

Below, I show this delta as a function of angle of incidence on the filter, for one filter at a time.

Filter 0 of 9: "B"-like

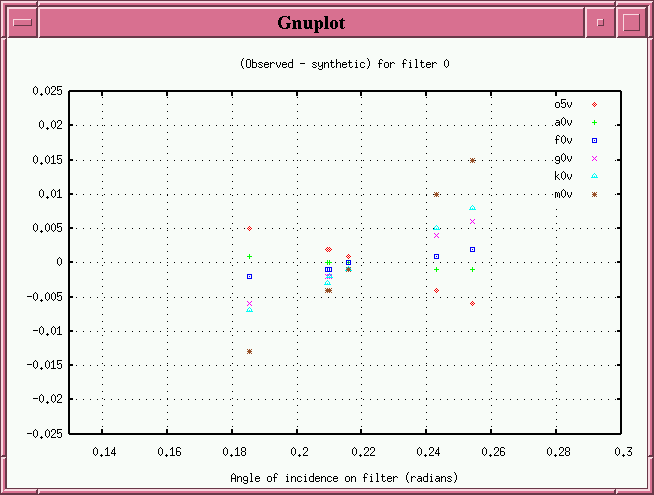
Filter 3 of 9: "I"-like

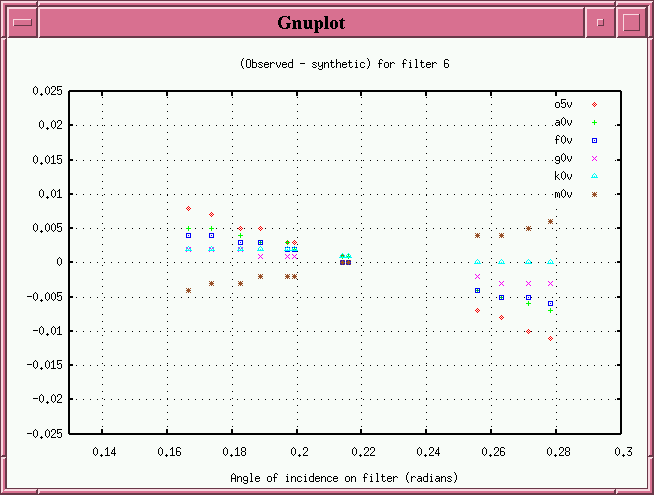
Filter 6 of 9: "near IR"

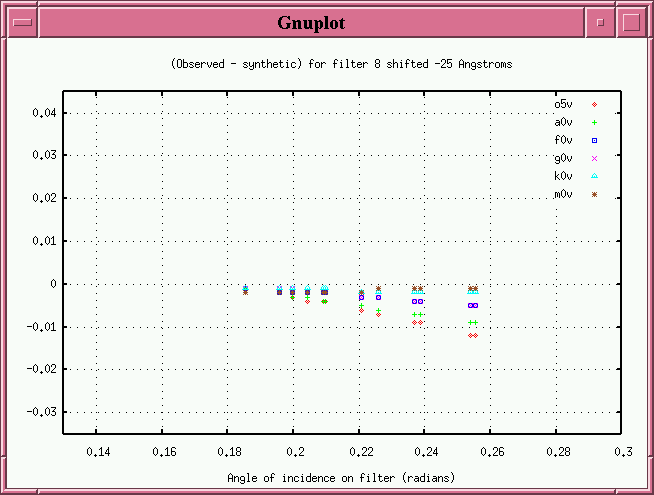
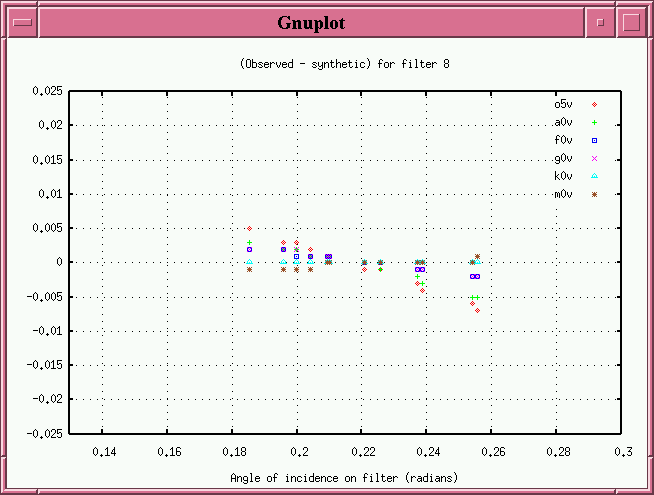
Filter 8 of 9: "far IR"

Notes:

• the sense of these changes in magnitude is always the same, but the size of the changes decreases in red filters
• this effect is smallest in hot stars (A,F) for the blue filters, but smallest in cool (G,K) in the red filters.

What is the effect of shift/error in filter transmission curve?

What if filters shift slightly after launch, so that the actual wavelengths are not equal to the design wavelengths, and we are not aware of this shift?

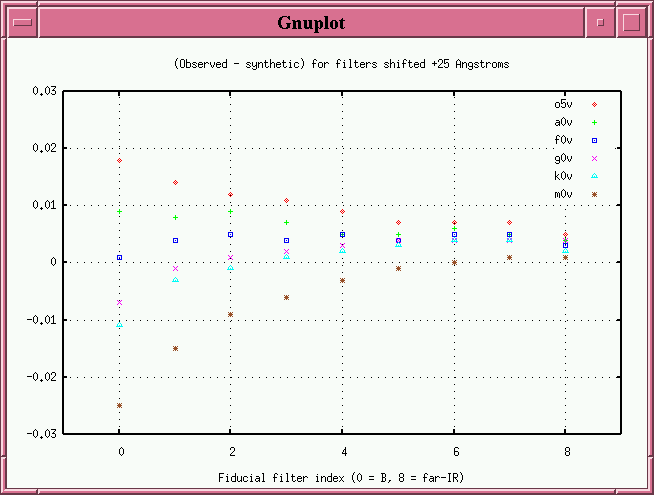
Below is a graph showing the errors in photometry, again in form delta = (observed - synthetic), for filters which have been shifted by +25 Angstroms.

Now, for filters which have been shifted by -25 Angstroms.

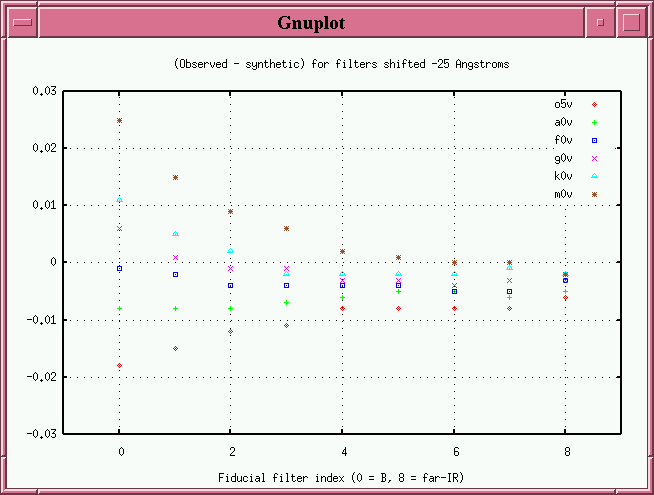
Notes:

• F and G stars suffer the smallest changes in photometry due to small shifts in filter passbands.
• these errors in photometry are largest in the blue filters, and much smaller in the near-IR

What is the effect of COMBINING shift/error in filter transmission curve with angle-of-incidence effect?

So, suppose that we now simulate a combination of effects:

• an unknown shift of -25 Angstroms in each filter bandpass
• the shift due to angle of incidence on the filter

Once again, I plot delta as a function of angle of incidence on the filter, for one filter at a time.

Filter 0 of 9: "B"-like

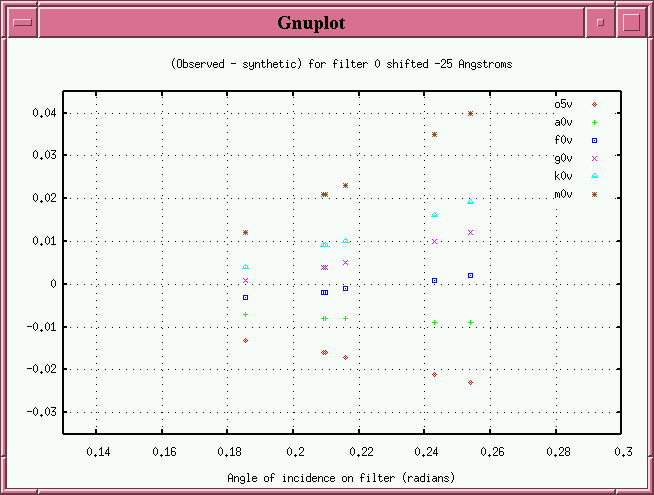
Filter 3 of 9: "I"-like

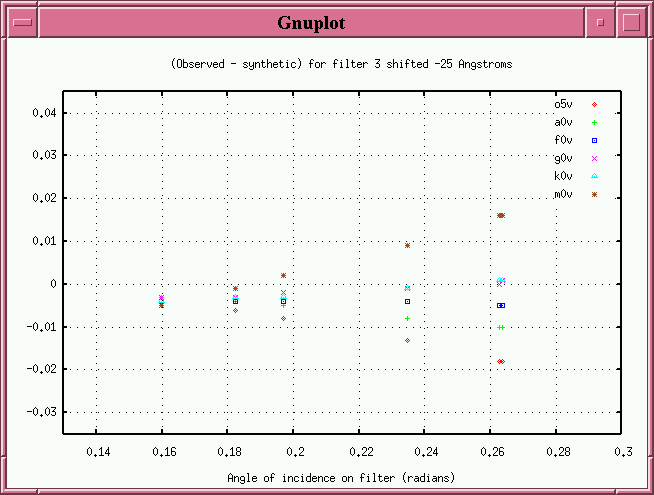
Filter 6 of 9: "near IR"

Filter 8 of 9: "far IR"