Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
On Earth, one way to describe a location is with a coordinate
system which is fixed to the Earth's surface.
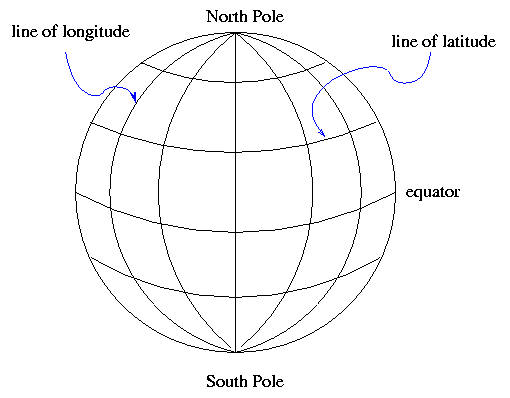
The system is oriented by the spin axis of the Earth,
and has special points at the North and South Poles.
We use lines of
latitude and longitude
to demarcate the surface.
It's obvious that latitude is measured away from the equator.
But where is the starting point for longitude?
There is no "obvious" choice.
After a lot of dickering,
European nations finally decided to use the
location of the Greenwich Observatory in England
as the starting point for longitude.
There are several ways to specify a location --
for example, that of the RIT Observatory.
One can use degrees:
Or degrees, minutes and seconds:
Or, in the case of longitude, one can measure in time zones.
The sun will set at the RIT Observatory about 5 hours and 11 minutes
later than it does at Greenwich,
so one could say
This ought to be pretty familiar. Think you understand it?
Then try applying your knowledge of latitude and longitude
to solve a simple little problem:
Finding the distance between two locations on Earth
is a first step in calculating the distance
to other objects in the solar system via parallax....
On can make a similar coordinate system which is "fixed
to the sky":
Once again, we use the Earth's rotation axis to orient the
coordinates.
There are two special places, the North and South Celestial
Poles.
As the Earth rotates (to the East),
the celestial sphere appears to rotate (to the West).
Stars appear to move in circles:
small ones near the celestial poles, and large ones close to the
celestial equator:
We again use two orthogonal coordinates to describe a
position:
Once again, there are several ways to express a location.
The star Sirius, for example, can be described as at
We can also express the Declination in Degrees:ArcMinutes:ArcSeconds,
just as we do for latitude;
and, as usual, there are 360 degrees around a full circle.
For Right Ascension, astronomers always use the convention of
Hours:Minutes:Seconds.
There are 24 hours of RA around a circle in the sky,
because it takes 24 hours for the Sun to move all the
way from sunrise to the next sunrise.
Thus, 1 hour of RA equals 15 degrees of RA.
Along the celestial equator, those 15 "degrees of RA"
are the same as 15 ordinary degrees.
That is, a star located at (RA = 6 hours, Dec = 0 degrees)
would be exactly 15 degrees away from a star located
at (RA = 7 hours, Dec = 0 degrees).
As one moves away from the celestial equator and toward the
poles, the lines of RA converge:
For points off the celestial equator,
the real distance in the RA direction is smaller
by a factor of cos(Dec).
Converting Sirius' position into sexigesimal form, we find
What's the difference between an "arcminute" and a "minute"?
You've learned trigonometry in high school:
sines, cosines, the Pythagorean Theorem, and all that jazz.
However, unless you went to a really good high school,
you probably restricted your calculations to planar geometry.
Unfortunately, the sky is not a plane. We measure
positions and coordinates on the inner surface of an imaginary
sphere.
That means that the old rules don't always work anymore.
The subject of spherical trigonometry is not a simple one,
but, in this course, we will only peek into it.
Given two vectors, a and b, what is the distance
between them?
On a plane, we can break up each vector into its components
and use the Pythagorean theorem:
Along the surface of the celestial sphere,
if we want to find the angular distance
between two points a and b,
we need to use the law of cosines.
In the usual case, the two points are expressed
in Right Ascension (α) and Declination (δ), like so:
In this case,
the law of cosines becomes
which gives us the cosine of the desired
angular distance γ.
If we are interested in very small angular distances
on the sky -- the separation between the two components of
a binary star, for example, or the distance between two
of the moons of Jupiter --
then there are two common approximations.
First, if we start with the RA and Dec coordinates of the two points,
we can make a pseudo-Pythagorean formula; all we have to do is
correct the difference in Right Ascension with the cosine
of the Declination.
Second, if we start with a picture of some very small region
of the sky, together with an indication of the scale
in arcseconds, like this:
then we can
Latitude and Longitude
latitude 43.0758 degrees North, longitude 77.6647 degrees West of Greenwich
latitude 43:04:33 North, longitude 77:39:53 West
latitude 43:04:33 North, longitude 05 hours 11 minutes West
Right Ascension and Declination
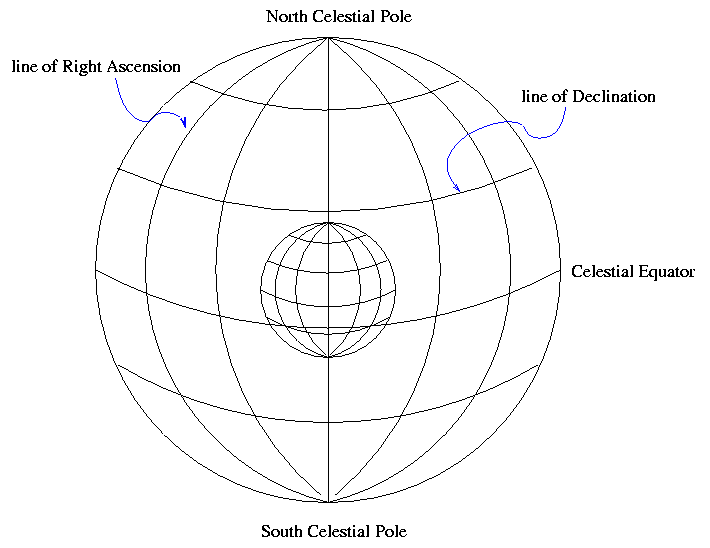
Image copyright
David Malin.
As with latitude, Declination is measured away from the celestial equator.
But there is again no obvious choice for the starting point of the
other set of coordinates.
Where should we start counting Right Ascension?
The rather arbitrary choice made by astronomers long ago was
to pick the point at which the Sun appears to cross the
celestial equator from South to North as it moves through the
sky during the course of a year.
We call that point the "vernal equinox".
Right Ascension 101.287 degrees, Declination -16.716 degrees
Q: What is the angular separation between
two stars at (RA = 6 hours, Dec = +60 degrees)
and (RA = 7 hours, Dec = +60 degrees)?
Right Ascension 06:45:09, Declination -16:42:58
A very little spherical trigonometry
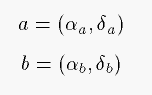
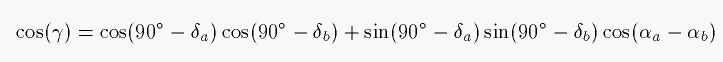
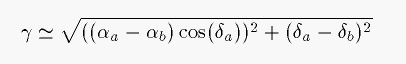
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.