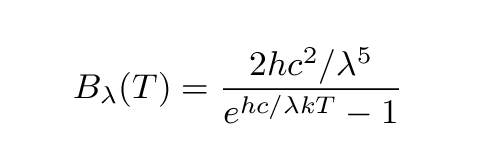
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
The total amount of energy radiated per second by a perfect blackbody depends only on its temperature T and area A:
4
energy per second = sigma * T * A
where
-8 Joules
sigma is the Stefan-Boltzmann constant = 5.67 x 10 ---------
s*m^2*K^4
T is the temperature of the object, in Kelvin
A is the surface area of the object, in square meters
This relationship is called the Stefan-Boltzmann Law.
If we consider a patch of area exactly one square meter, then the energy radiated from it per second is
4
energy per second = sigma * T
per square meter
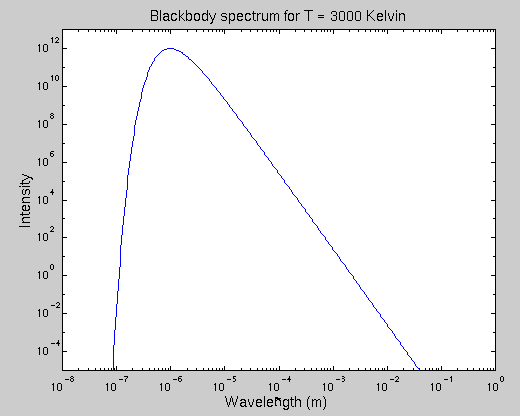
A blackbody doesn't emit equal amounts of radiation at all wavelengths; instead, most of the energy is radiated within a relatively narrow band of wavelengths. The location of that band varies with the body's temperature; for example,
object T (Kelvin) radiates mostly
------------------------------------------------------------------------
very cold gas in insterstellar space 20 radio
a live human being 310 infrared
the Sun 5,600 visible
interior of nuclear explosion 3,000,000 X-rays
The exact amount of energy emitted at a particular wavelength lambda is given by the Planck function:
(2*h*c^2) / lambda^5
B (T) = ----------------------
lambda h*c/lambda*k*T
e - 1
Or, in beautiful typeset format,
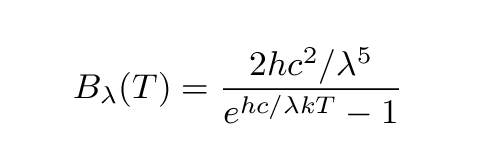
In this equation,
B (T) is the energy (Joules) emitted per second per unit
lambda wavelength per steradian from one square meter
of a perfect blackbody at temperature T
T is the temperature of the blackbody
h is Planck's constant = 6.63 x 10^(-34) J*s
c is the speed of light = 3.00 x 10^(8) m/s
lambda is the wavelength
k is Boltzmann's constant = 1.38 x 10^(-23) J/K
The Planck function has a distinctive shape: it rises very sharply at short wavelengths (due to the exponential), reaches a peak at some wavelength, then falls gradually at longer wavelengths.
In order to find the total energy emitted per second in some wavelength range
lambda 1 < lambda < lambda 2
from one square
meter of a perfect blackbody,
one can integrate the Planck function
lambda 1
/ (2*h*c^2) / lambda^5
energy emitted = | ---------------------- d(lambda)
per second / h*c/lambda*k*T
per square meter lambda 2 e - 1
per steradian
and then multiply by (pi) steradians (we are interested in the energy emitted at all angles from one face of a flat plate, so we integrate over solid angle to cover half the sky; that ends up being the same as multiplying by a factor of (pi) = 6.28...)
Or, in nicely typeset form,
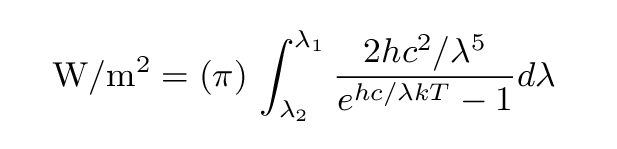
Obviously, if one integrates from the shortest possible wavelength (lambda = 0) to the longest possible wavelength (lambda = infinity), and multiplies by (pi), one ought to end up with the same total energy emitted per second as given by the Stefan-Boltzmann Law,
4
energy per second = sigma * T
per square meter
Last modified 4/06/2007 by MWR.
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.