
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
First, consider the tradeoff between describing accurately the wavelength of a wave and its position.
So there appears to be a tradeoff: one can measure the wavelength precisely by looking at a long string of sensors -- but then the position of the wave is pretty loose. If we want to know the position of a sharp jolt, we pay attention only to a short string of the sensors -- but then the wavelength is hard to calculate. One can put the tradeoff into a mathematical form like so:
(delta wavelength) * (delta position) ~ const
where delta is short for "uncertainty in the measurement of."
The usual form of this relationship replaces wavelength by its inverse, wave number k:
2 * pi
wave number k = ----------------
wavelength
Stated this way, the relationship looks like:
(delta wavenumber) * (delta position) ~ 1
The constant factor here may not be exactly 1.0, but it is of that order; closer to 1 than it is to 100 or 0.01.
De Broglie showed that particles of matter exhibit wave-like properties under certain circumstances, with wavelengths which depend on the particles' momentum:
h
wavelength = -----------
momentum
Or, using wavenumber k instead of wavelength,
2 pi h
---------- = -----------
k momentum
which leads to
h * k
momentum p = -----------
2 pi
Heisenberg realized that this meant that particles -- like any waves -- would have to obey the tradeoff between wavelength and position. Since wavelength and momentum are simply connected, this meant that one could turn the classical equation for waves
(delta x) * (delta k) ~ 1
into one for matter:
(delta x) * h * (delta k) ~ h * 1
(delta x) * (delta p) ~ h
With a careful analysis, Heisenberg was able to show that the constant factor was not quite Planck's constant, but a bit less.
h
(delta x) * (delta p) ~ --------
2 * pi
Note that in some textbooks, this product of uncertainties is not exactly h/(2 π), but half that:
1 h
(delta x) * (delta p) ~ - --------
2 2 * pi
The coefficient out in front of this expression is a matter of some debate. It's very difficult to perform experiments in which complementary properties such as position and momentum can both be measured accurately enough to reveal the value of this product to two decimal places. Don't worry: as long as you remember that the product is some small fraction of Planck's constant h, you'll be fine.
So Planck's constant turns out to govern not only
but also
It turns out that one can do a little algebraic manipulation to Heisenberg's Uncertainty Principle to change the related quantities: from position and momentum to energy and time. Look:

Heisenberg had some strong words on how one should interpret the arcane mathematical equations which began to fly around in the nineteen-teens and -twenties.

He came up with a "thought experiment" which illustrates the physical connection between a particle's position and momentum; or, rather, with our ability to measure them.

Image courtesy of
the AIP History
site on Heisenberg
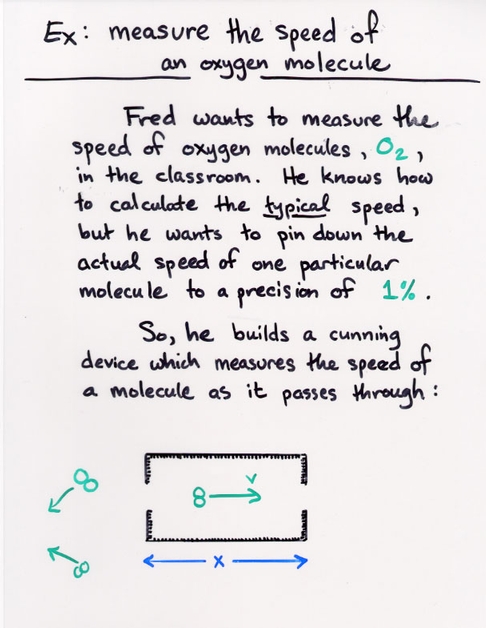
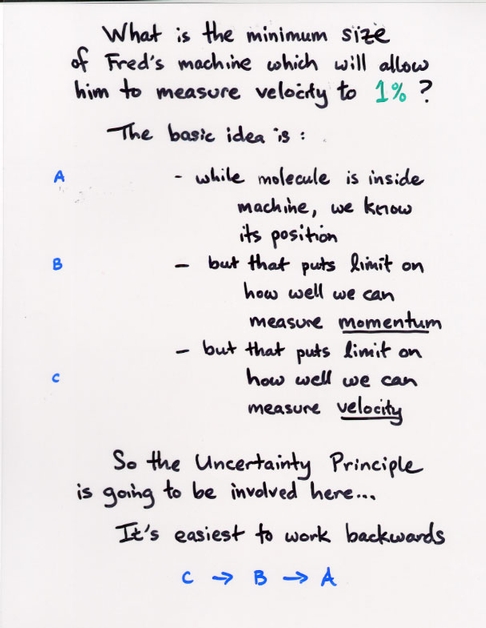
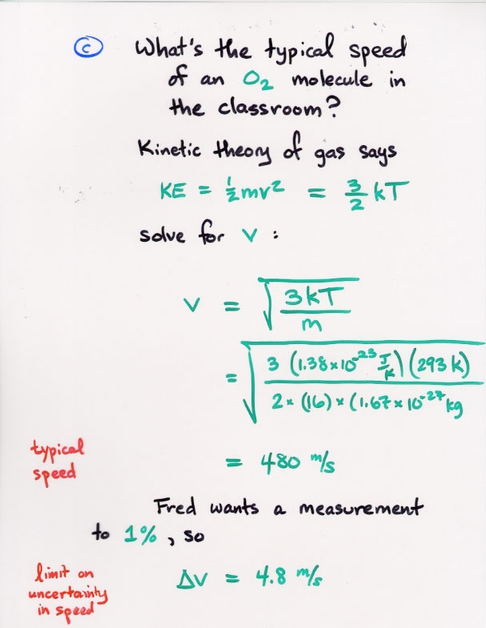
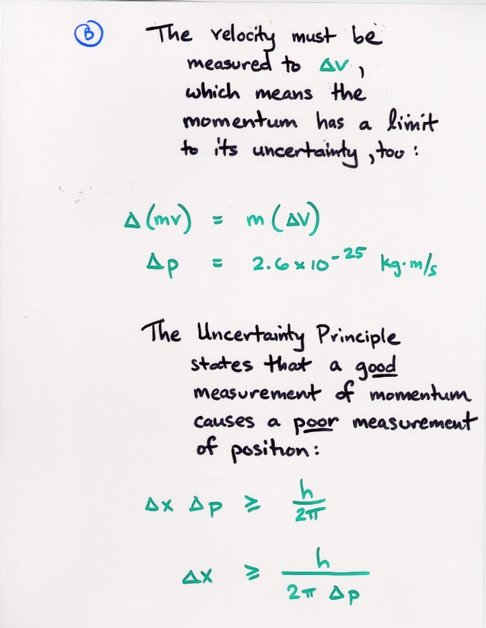

 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.