Using diffraction gratings: laser light and digital media

Your job is to use a diffraction grating to measure

• the wavelength of a red laser (again)
• the spacing of grooves in a CD and/or DVD

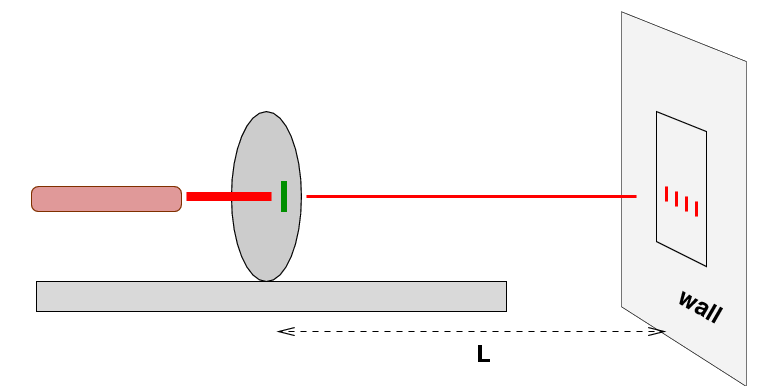
The first involves the same procedure you've used in the recent past: shine laser light through a device and measure the separation of bright or dark spots on a distant screen:

Set up an optics bench and laser as shown above. Instead of shining the laser through a wheel, shine it through a diffraction grating which sits on an optics component carrier. Place a piece of paper on the wall, and arrange things so that you can mark on a piece of paper the central bright spot and two spots to the side. The distance L will probably be smaller than you've used in the past -- perhaps 50 or 60 cm. It might help to tape two or three pieces of paper together.

1. Measure the separation between the central spot and the first two spots to the side.
2. Make a table of your measurements, which will be the distances L and y. Each value must have units and an estimate of uncertainty.

3. Now, compute the wavelength of the light. Just use a simple formula to find the wavelength. You must also propagate the uncertainties in your measured distances to determine the uncertainties in this wavelength.

4. Does your result from the first spot agree (within uncertainties) with your result from the second spot?

If all went well, your value for the wavelength of laser light should be similar to, but more precise than, your earlier measurements.

The spacing of tracks on a CD and/or DVD

The goal is to determine the spacing between the tracks (or grooves) on the surface of a CD or DVD. This time, you must figure out the procedure. You should adopt the wavelength of laser light from your work above.

• taping sheets of paper together to make a very wide piece of paper might be a good idea
• make sure you draw a clear diagram showing the setup of your equipment, and the path of light of the laser
• make a table showing all your measurements, including all distances
• compare your results with those from other groups: are your values the same within the stated uncertainties?
• do CDs have the same track spacing as DVDs? If not, does the difference in track spacing explain the difference in storage capacity? Be quantitative!

Copyright © Michael Richmond. This work is licensed under a Creative Commons License.