Collisions in two dimensions

All the examples you have considered so far involve motion in a single dimension, along a track or a road. What happens when objects are free to move and bounce in two or three dimensions?

It turns out that momentum is (in the absence of external forces) conserved in each direction individually. In other words,

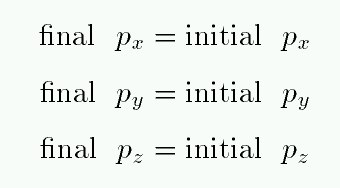
or, in more compact notation,

Example of a two-dimensional collision

Use your computers to analyze a video in which two pucks collide on an air table. You might want to read the instructions for marking properties on videos in LoggerPro.

1. Go to the "Student Shares" -> ... "LoggerPro" -> "CofM Video" folder.

2. Double-click on the 1D_CoM_P017 file. It shows a collision between two pucks of different mass on an air table.
   1. What is the momentum of each puck in each direction (x, y) before the collision?
   2. What is the magnitude of the momentum of each puck before the collision (i.e. the combination of x and y components)?
   3. What is the total momentum in each direction before the collision?
   4. What is the momentum of each puck in each direction (x, y) after the collision?
   5. What is the magnitude of the momentum of each puck after the collision?
   6. What is the total momentum in each direction after the collision?
   7. Is the total momentum conserved in each direction? If not, there must be some external forces acting on the pucks. What are the most likely suspects?
   8. If you add the magnitudes of the momentum of each object before the collision, and then after the collision, do you find the same value? Why not?

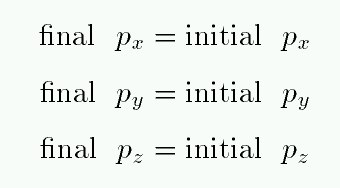
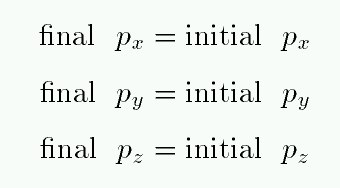
   Copyright © Michael Richmond. This work is licensed under a Creative Commons License.