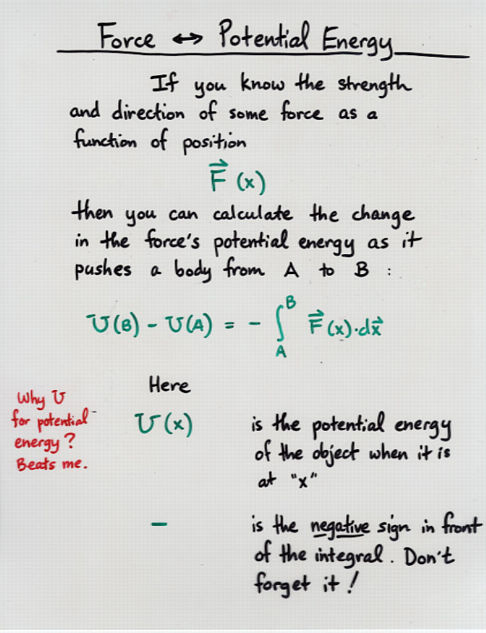
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Not all forces have associated potential energies. For example,
We call forces which do have associated potential energies conservative forces. One way you can recognize a conservative force is to take an object on a round trip and see what happens to it.
Conservative Non-conservative
----------------------------------------------------------------------
Examples gravity friction
spring force air resistance
electricity water resistance
Work done by
force during
a round trip zero negative
(closed path)
Does it have
a potential energy? yes no
----------------------------------------------------------------------

Here's a somewhat more sophisticated map showing potential energy -- electric potential energy in this case -- as a function of position on a piece of paper.
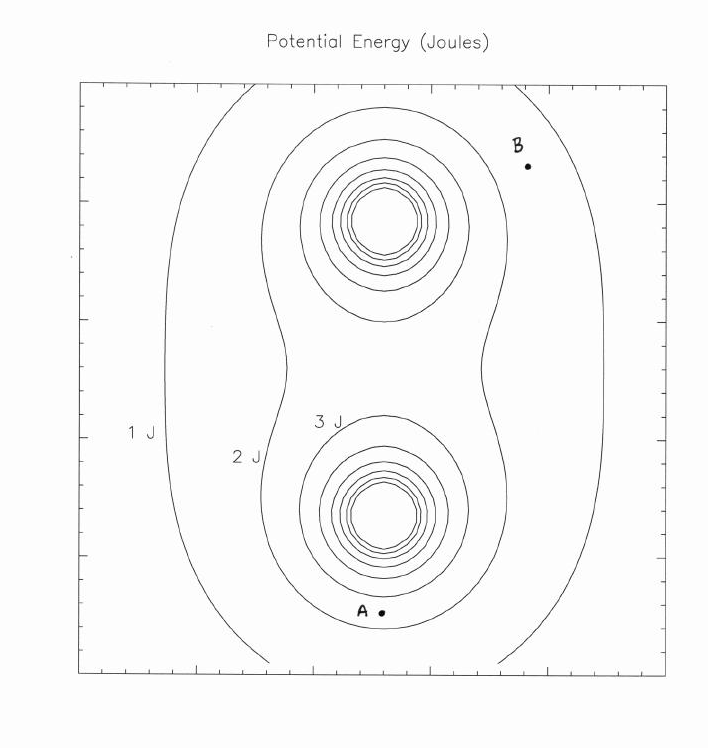
Q: What is the change in potential energy
per meter at location A, if one
moves to the right on this map? If one moves
downwards on this map?
Q: What is the change in potential energy
per meter at location B, if one moves
to the right on this map? If one moves
downwards on this map?
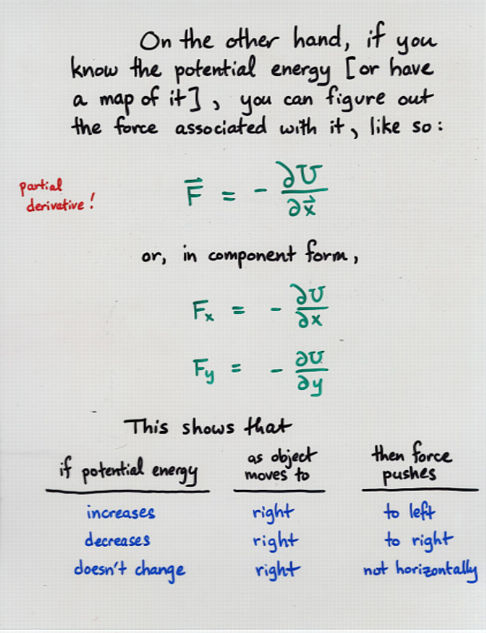
Q: What is the size, and direction, of the
force on a particle at point A?
Express this force in unit-vector notation.
Q: At what locations is the total force on
a particle zero? At which of these places
is there a stable equilibrium?

Suppose that in some region of space, the potential energy U (measured in Joules) is a function of position (x, y) (measured in meters) like this:
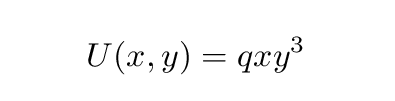
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.