Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
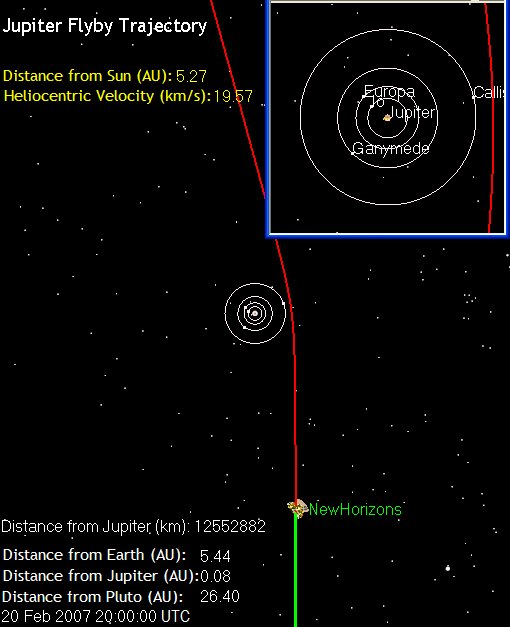
Right now, on Wednesday, Feb 21, 2007, the New Horizons spacecraft is approaching Jupiter:
Even though the spacecraft is still over 10 million kilometers from Jupiter, it is taking very nice pictures of the Jovian weather patterns.
However, this is just a warm-up for the New Horizons spacecraft. Its real target is much, much, farther away: PLUTO. The spacecraft must be patient -- it's going to take a long, long, lo-o-o-o-ng time to get there. It is not scheduled to reach Pluto until the year 2015!
If we look at the current information from the New Horizons web site, we can see the current speed of the spacecraft:
That's pretty fast. But as it moves farther and farther from the Sun, the gravitational force between the Sun and the spacecraft will perform more and more negative work, slowing the ship down. How fast will it be going when it reaches Pluto in 2015?
Well, we could try using conservation of energy ....
Now: R = 5.27 AU, v = 19,600 m/s, m = 480 kg
KE =
GPE =
total E =
In 2015: R = 32.83 AU, m = 480 kg
total E =
GPE =
KE = ????
so v = ????
Now, if we actually look at the mission plan, it turns out that the New Horizons spacecraft will be travelling much faster when it passes Pluto and Charon: v = 13,800 m/s. How can that be?
Then how can it pick up this extra speed and kinetic energy? The answer is -- theft.
If you look closely at the mission trajectory, you'll see that when the spacecraft passes Jupiter, its direction changes:
The gravitational forces between Jupiter and the spacecraft end up adding a new component to the spacecraft's velocity.
How does this work? Let's take a simpler collision as an example.
Joe stands by the side of the road and (very stupidly) tosses a tennis ball of mass m = 0.1 kg towards the road. As the ball hangs in mid-air, a giant truck of mass M = 10,000 kg smashes into it at speed V = 30 m/s.
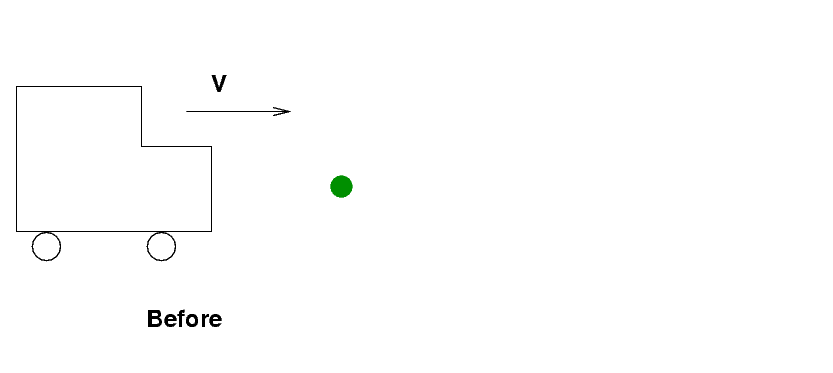
After the collision, the ball flies forward at speed V2 down the road, while the truck continues on its way with some (slightly smaller) speed V1.
If this is an elastic collision, so that both momentum AND kinetic energy are conserved, can you figure out what the final speeds are? Hint -- see section 9-10 of your textbook, around page 221.
The answer is that if a very massive object smashes into a low-mass object which is at rest, the very massive object slows down just a teeny-tiny bit, while the low-mass object zooms ahead at about twice the speed of the massive object. A very small amount of the original KE and momentum of the massive object is transferred to the low-mass object.
Something very similar is happening with the New Horizons spacecraft and Jupiter. Jupiter is majestically orbiting around the Sun at a speed of about 13,100 m/s. As the spacecraft slips behind Jupiter, the gravitational force of Jupiter pulls New Horizons forward. Because the spacecraft doesn't come _too_ close to the planet, only a portion of the maximum possible energy and momentum is transferred to the spacecraft: it picks up a transverse velocity of about 9700 m/s to go with its original radial velocity of about 19,600 m/s.
So, when the spacecraft reaches Pluto, it will be moving at about 13,800 m/s. At its closest approach, the spacecraft will be only about 10,000 km from the planet's surface. Roughly how long will it take the spacecraft to zip past the planet?
That's not very long -- it doesn't give the scientists much time to study the planet. Why doesn't the spacecraft use its rocket engines to slow down? It would be really nice to slow down enough to enter an orbit around Pluto -- that would require a final speed of about 800 m/s.
Suppose exhaust speed is u = 2,600 m/s Mass of spacecraft m = 480 kg Initial speed v = 13,800 m/s Final speed vf = 800 m/s What would initial mass of the (spacecraft + fuel) have to be to yield this change in velocity?
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.