Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
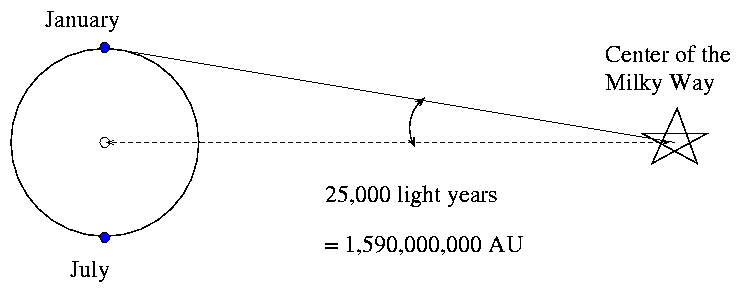
If you look in your textbook on page 309, you'll see a diagram of the Milky Way Galaxy. It indicates that the disk is about 75,000 light years across, and the Sun is about 25,000 light years from the center of the bulge. How do we know these dimensions?
Is it possible to use parallax to measure the distance to the center of the Milky Way? The best parallax measurements from the Hipparcos satellite are around 0.003 arcseconds, or 8 x 10^(-7) degrees. Let's see ....
Q: How large would the parallax angle be?
Hmmmm. Parallax won't do the job.
You can observe clusters of stars, and use the HR diagram of each cluster to estimate its distance. But what if you want to know the distance on a finer scale, in between clusters?
In some circumstances, astronomers can use individual stars as standard candles to measure distances across the galaxy. A "standard candle" is simply an object of known luminosity. If we know the total power emitted by a star, or, equivalently, we know its absolute magnitude, then it may be used as a standard candle. Finding distances with standard candles is easy:
So, all we need is some type of star we can use as a standard candle. Fortunately, Nature has provided two types of stars which are easy to recognize, and easy to use.
If you take a single picture of a distant cluster of stars, you see ... a bunch of stars.
You can measure the brightness of each star in the cluster. Then you have a bunch of numbers ....
But if you take a second picture of the cluster, and then a third, and then another, and another, and another, and measure the brightness of all the stars in all the pictures, THEN you discover something interesting:
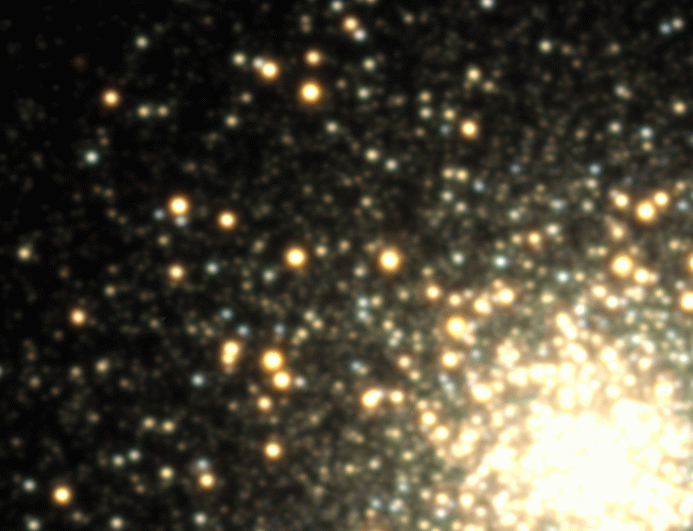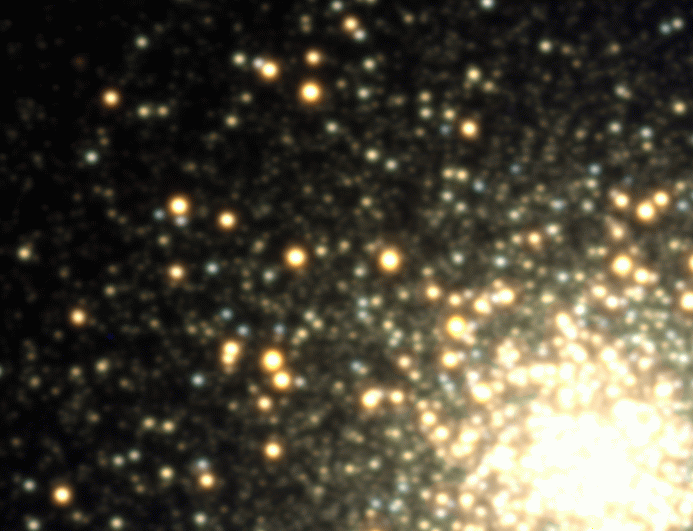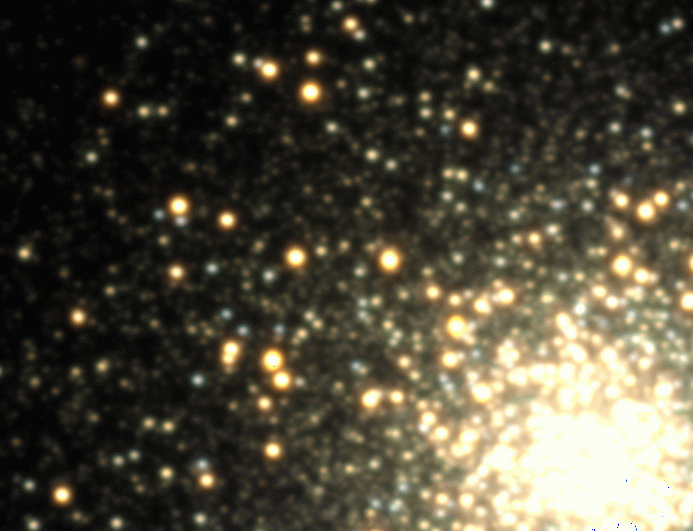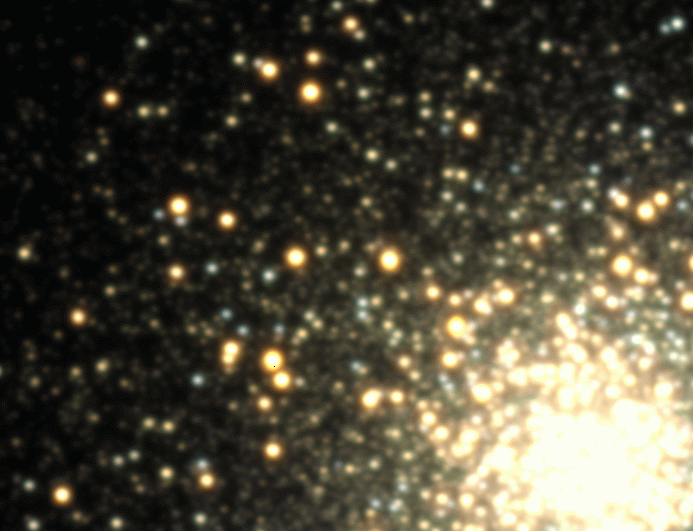
Image courtesy of
J. Hartman
and
K. Stanek
( Harvard CFA )
Most of the stars remain the same brightness all the time, but a small fraction vary, growing brighter and fainter, brighter and fainter.
Many of these variable stars have a set of common features:
We call this class of variable star RR Lyraes, after one particular member, the star RR in the constellation of Lyra.
Oh, and one more thing they have in common: they all produce roughly the same power. Look at the magnitudes of a set of RR Lyrae stars in a cluster called Draco (taken from a paper by Bonanos et al. ):
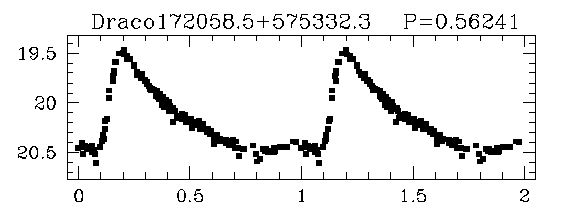
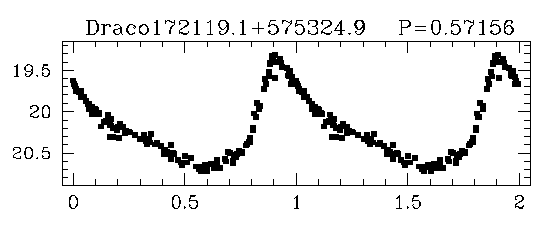
If they emit the same amount of light, then we can use them as standard candles. All we need to do is find out their absolute luminosity, and then we're set. It turns out that RR Lyrae stars are significantly brighter than the Sun. To a rough approximation, we can say they all have
RR Lyrae stars: (average) absolute mag M = 0.6
Their colors vary as they pulse, but typically lie between (B-V) = +0.20 and (B-V) = +0.50.
Q: Where do RR Lyrae stars fall on the HR diagram?
So, using this absolute magnitude, and the equation on page 312 of your textbook, can you figure out how far away from Earth the star RR Lyrae is?
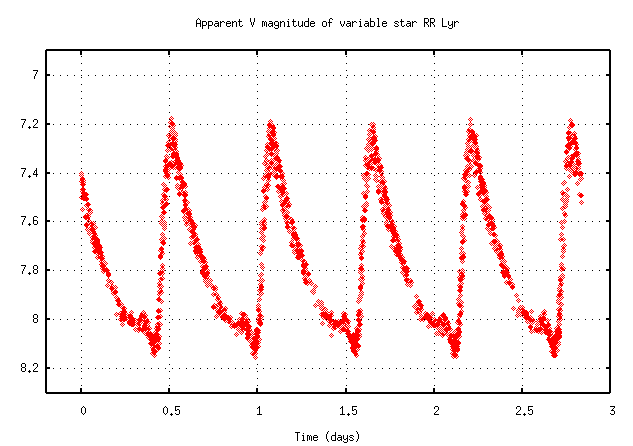
Cepheids are another class of variable stars which may be used as standard candles. They are both better and worse than RR Lyrae stars:
Hey! How can we use Cepheids as standard candles if they don't all have the same luminosity? Well, take a look at this diagram; it shows the apparent magnitude of stars in a distance cluster called the LMC, plotted as a function of their period.
Thanks to the MACHO group
Notice two things:
However, there is a saving grace: the absolute magnitude of a Cepheid variable star is correlated with its period. Longer periods mean more powerful stars. This is especially clear if one plots the magnitude of stars against the logarithm of their periods:
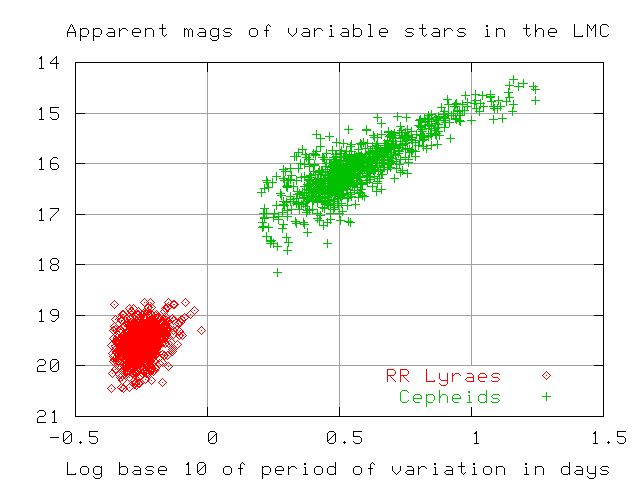
It is possible to make a simple equation which will predict the absolute magnitude of a Cepheid star, given its period. One analysis suggests
Cepheids: (average) absolute V mag M = -1.0 - 2.8 * log(Period)
The colors of Cepheids cover quite a range, due to their wide range of masses and their variations as they pulse; to a rough approximation, they span (B-V) = +0.5 to +1.0.
Q: Where do Cepheid stars of period P = 10 days fall on the HR diagram? Q: Where do Cepheid stars of period P = 100 days fall on the HR diagram?
So, all you have to do is
It's a bit more work than for RR Lyrae stars, but since Cepheids are brighter, you can use them to measure greater distances.
Give it a try: below are some measurements of one Cepheid star (delta Cephei, the prototype of its class). Can you figure out its distance away from the Earth?
So, how can we use these variable stars to find the size of the Milky Way? Suppose that we want to measure the distance between the Earth and the center of the galaxy. All we have to do is take pictures of the center of the Milky Way, find some Cepheids or RR Lyrae stars, and ...
We can't see ANYTHING at the center of the Milky Way! Clouds of dust and gas block our view!
So what can we do?
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.