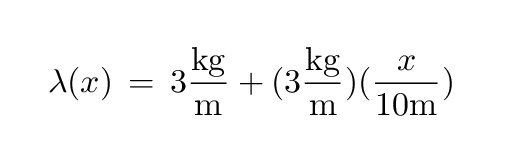Computing the moment of inertia of an extended body

For example, let's consider a long, thin rod of uniform density, with mass M and length r. The rod is is attached to a spinning hub at its left end.

What we need to do is to break the rod into tiny little compact pieces ... because we know how to compute the moment of inertia of one compact little piece.

In fact, we need to break the rod up into an INFINITE number of VERY SMALL pieces; and that means it's time for calculus.

Okay, your turn. What happens if the density of the rod isn't uniform, but changes from one end to the other? Suppose that a rod has length L = 10 m and density

1. What is the total mass of this rod? (Hint: integrate the mass of tiny pieces from one end to the other)
2. What is the moment of inertia of this rod? (Hint: integrate the moment of inertia of tiny pieces from one end to the other)

For more information

• Table of moments of inertia for selected bodies (PDF) (If the PDF doesn't look good, try a copy in PNG )
• Step-by-step example: calculating the moment of inertia of a disk (PDF)