Theory of Damped Harmonic Motion

The general problem of motion in a resistive medium is a tough one. We will make one assumption about the nature of the resistance which simplifies things considerably, and which isn't unreasonable in some common real-life situations.

Start with an ideal harmonic oscillator, in which there is no resistance at all:

We could write the equation this way ...

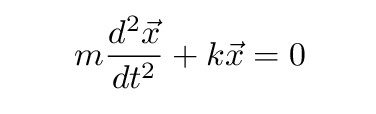
You know the solution to this equation, right?

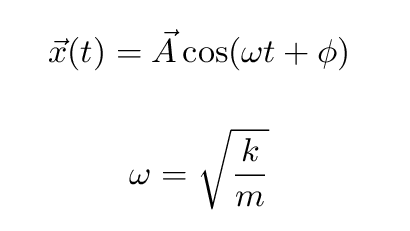
Right. The important points of this method of analysis are:

we have an equation which provides the position of the object as a function of time
the equation include a parameter -- in this case, the angular frequency ω -- which depends on the coefficients in the differential equation -- in this case, k and m

Okay, now let's add some resistance. We'll make the assumption that the resistive force

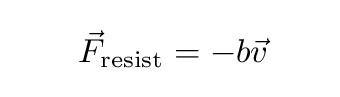
is always opposite to the direction of motion (i.e. opposite to the velocity)
depends LINEARLY on the magnitude of the velocity

Is this always true? No. Is it sometimes true? Yes --- to some degree. For example, when objects move slowly through the air, at just a few meters per second, the force of air resistance grows roughly with speed in a linear fashion.

If we make this assumption, then the sum of forces on the object becomes

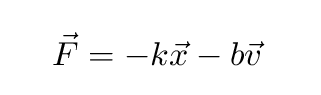
We can write everything in terms of position x(t) and its derivatives:

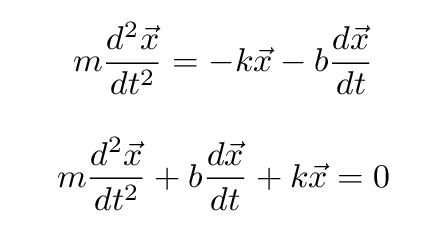
Uh-oh. This could be trouble. How can we solve this differential equation? The goal is again to come up with

an equation which provides the position of the object as a function of time
an equation which includes parameters which depend on the coefficients in the differential equation -- in this case, k and m AND b

The answer is (in my case, at least), take a guess ... but make it an educated guess. I know that solutions to the simpler differential equation without the velocity term look like sines and cosines; and solutions to the simpler differential equation without the acceleration term look like exponential functions.

Plus, if I just watch the motion of a real mass attached to a real spring, and wait for a while, I can see that the motion gradually dies down ...

So, I'm going to GUESS that the solution to this big differential equation will be some sort of combination of those two solutions. (I'm going to drop the vector notation at this point, because we're going to have enough to deal with as it is ...) So we GUESS

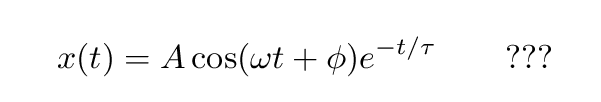
Notice that this equation has two parameters:

ω something like an angular frequency
τ something like a time constant

If all goes well, we'll be able to relate these parameters to the coefficients of the differential equation: k, m and b.

So, what do we do? Well, we've made our GUESS .... and now it's time to CHECK the result.

Please write down the first derivative of this function. Then write down the second derivative of this function. It may take a while -- that's okay.





            Go ahead and write down the first derivative.  I'll wait ....






            Now write down the second derivative.  I'll wait ....

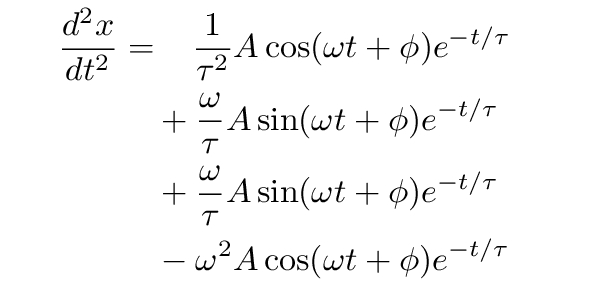
Phew.

Now, go back to the equation of motion for the object:

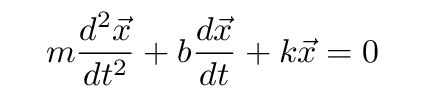
In order to verify that our GUESSED solution actually does satisfy this equation, we need to insert the full expressions for the original function, its first derivative, and its second derivative ... and then make sure that it all adds up to zero. Let's do that ...







    much pain and agony later ....

Yes, our guessed solution will satisfy the equation as long as

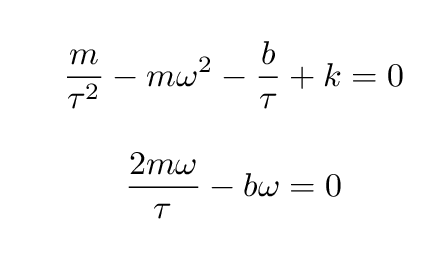
So, if we can measure the mass m, and the force constant k, and the resistance force coefficient b, then we can compute

the time constant τ
the frequency of oscillation omega

Please solve for τ AND omega in terms of the other variables now.

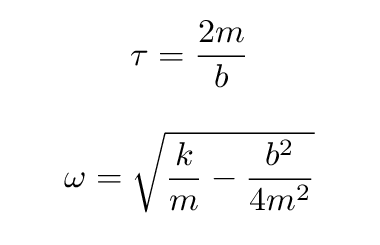
Well, fine, we have solved some equations. But what do they MEAN?

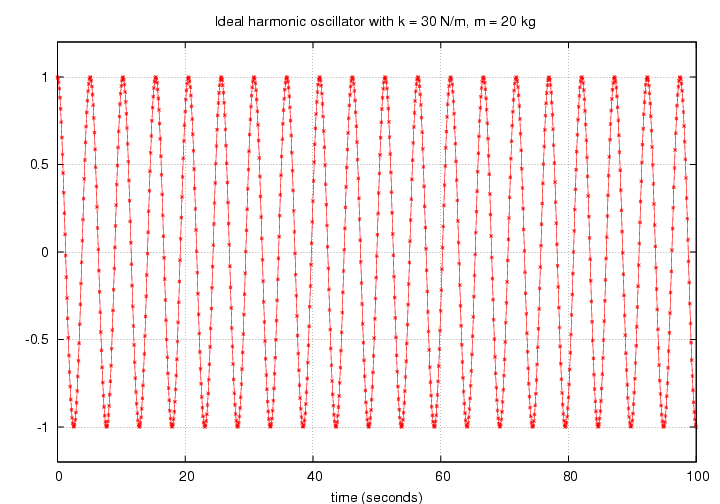
The ordinary harmonic oscillator moves back and forth forever. It converts kinetic to potential energy, but conserves total energy perfectly. It will never stop.

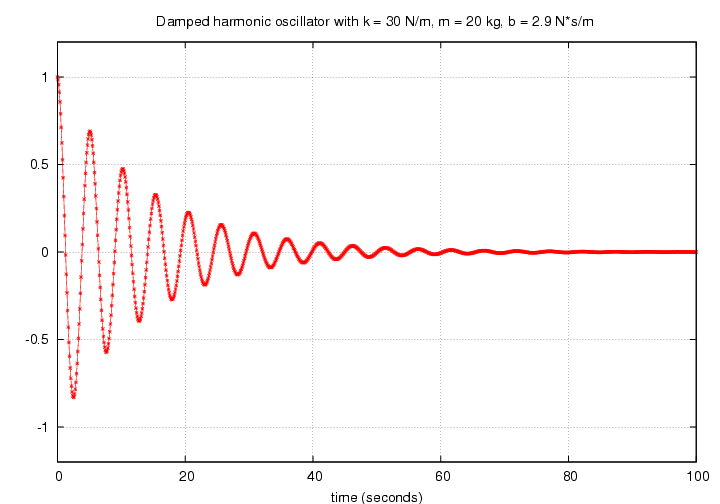
A lightly damped harmonic oscillator moves with ALMOST the same frequency, but it loses amplitude and velocity and energy as times goes on. The timescale over which the amplitude decays is related to the time constant τ .

As the resistive force increases (b increases), the decay happens more quickly.

When the combination of parameters is just right, critically-damped so that

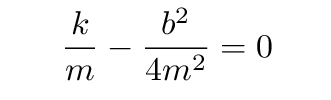
then the oscillator never really oscillates: it decays exponentially, just barely not reaching the other side of the equilibrium.

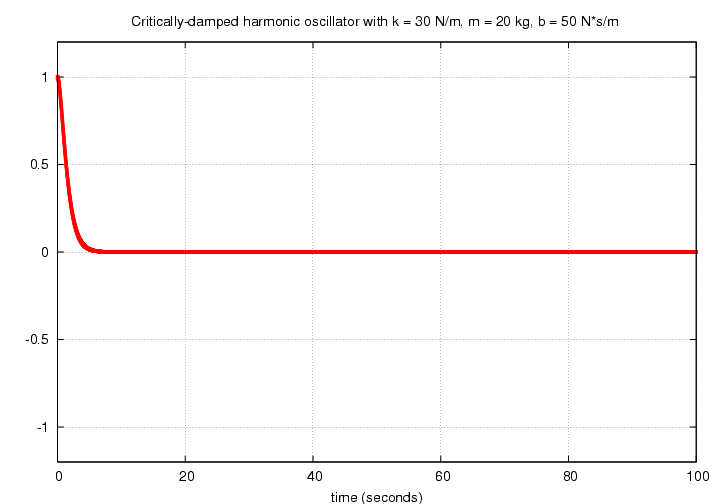
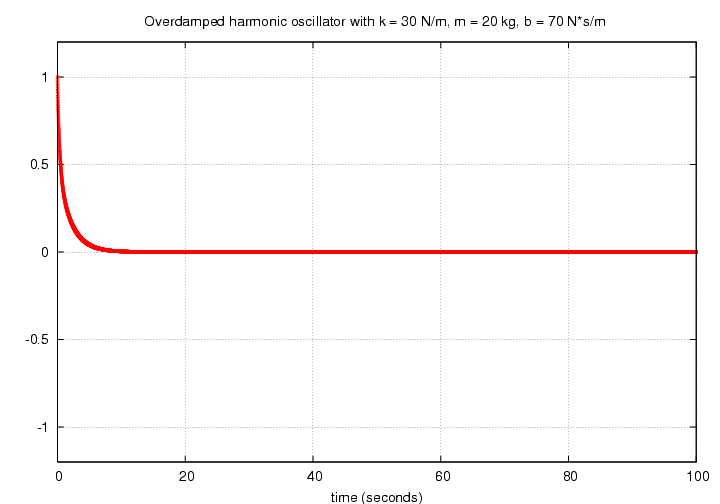
If one makes the resistive force even stronger, the over-damped oscillator decays in an even quicker manner.

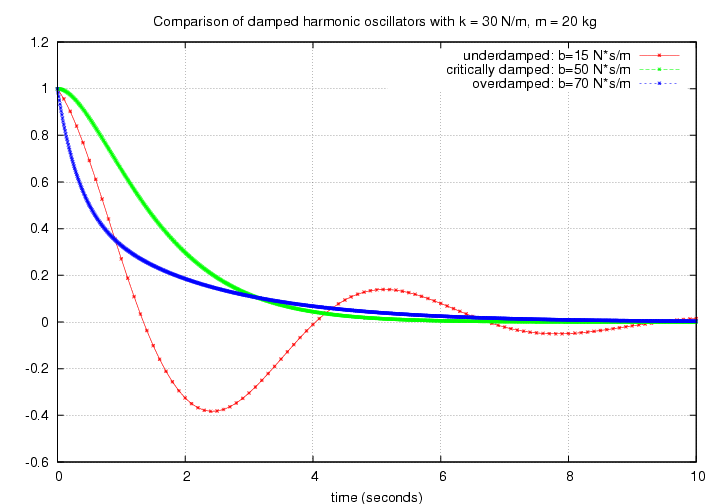
Let's look at the first few seconds of an under-damped, critically-damped, and over-damped case so that we can see the initial motions more clearly.