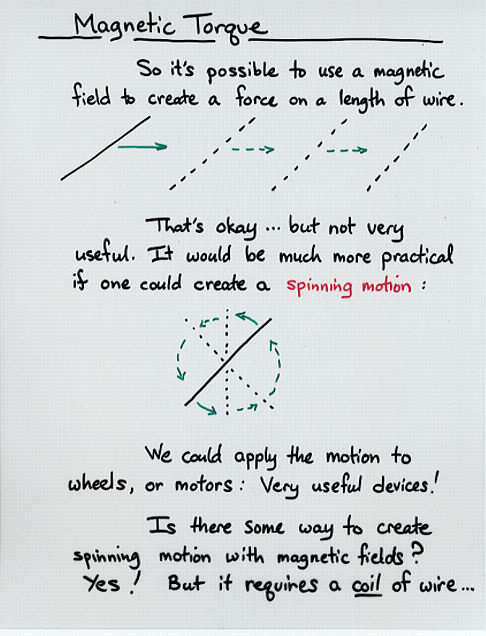
 Viewgraph 1
Viewgraph 1 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
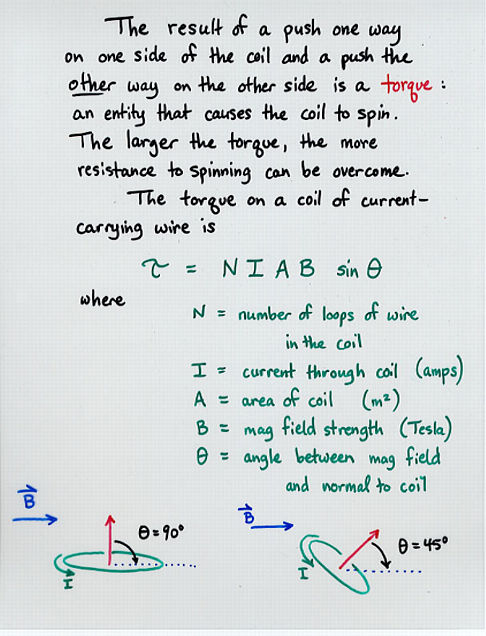
torque = (# turns) * (current) * (loop area) * (mag field) * sin(theta)
where theta is the angle between the magnetic field and the loop's
normal vector.
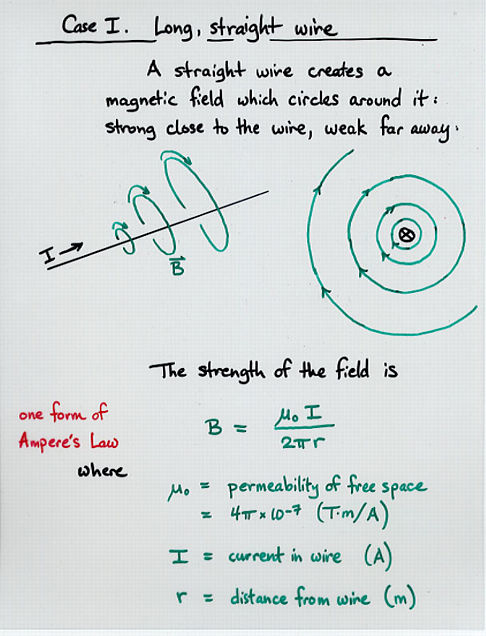
mu * current
mag field = -------------------
2 * pi * distance
where mu is the magnetic permeability of free space:
mu = 4 * pi * 10^(-7) T*m/A
= 1.257 x 10^(-6) T*m/A
N * mu * current
mag field = -----------------
2 * radius
where N is the number of turns of wire in the loop.
 Viewgraph 1
Viewgraph 1
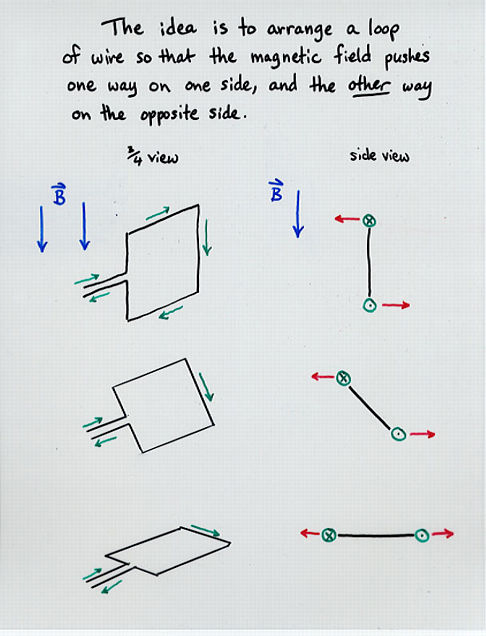 Viewgraph 2
Viewgraph 2
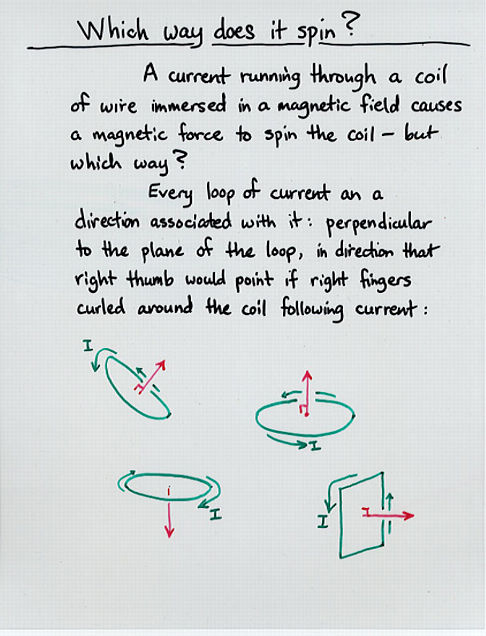 Viewgraph 3
Viewgraph 3
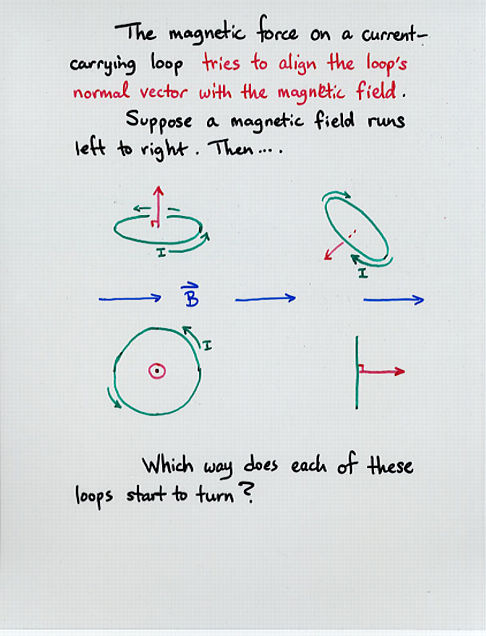 Viewgraph 4
Viewgraph 4
 Viewgraph 5
Viewgraph 5
 Viewgraph 6
Viewgraph 6
 Viewgraph 7
Viewgraph 7
 Viewgraph 8
Viewgraph 8
 Viewgraph 9
Viewgraph 9
 Viewgraph 10
Viewgraph 10
 Viewgraph 11
Viewgraph 11
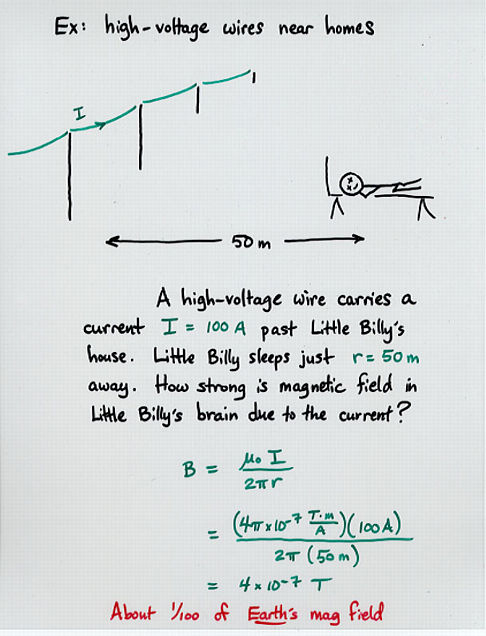 Viewgraph 12
Viewgraph 12
 Viewgraph 13
Viewgraph 13
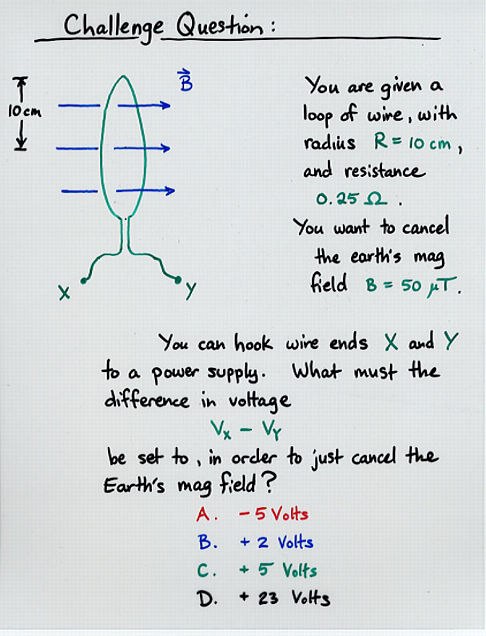 Viewgraph 14
Viewgraph 14
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.