Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
You remember linear momentum, right? Suppose that an object of mass m is moving in some direction with velocity v.
The object will continue moving in the same direction, with the same speed .... unless some external force perturbs it. One way to describe this tendency of objects to continue moving with a constant velocity is that they contain linear momentum,
The larger an object's linear momentum, the larger a force must be to perturb its motion. We can describe this relationship mathematically with a very familiar equation.
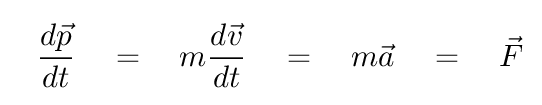
Okay, now let's move into the ANGULAR realm.
Suppose that an object with moment of inertia I is spinning in some direction with angular velocity ω.
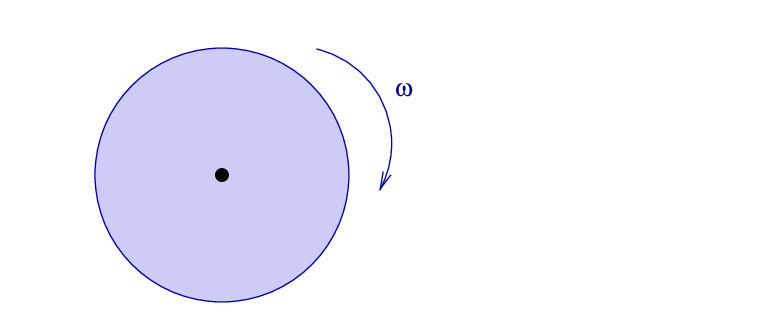
The object will continue spinning in the same direction, with the same angular speed .... unless some external torque perturbs it. One way to describe this tendency of objects to continue spinning with a constant angular velocity is that they contain angular momentum,
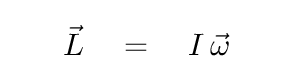
The larger an object's angular momentum, the larger a torque must be to perturb its motion. We can describe this relationship mathematically with a new equation:
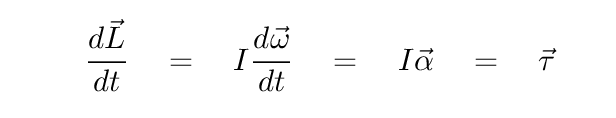
What are the units of angular momentum? Let's try to work it out.
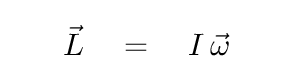
Q: What are the units of moment of inertia I? Q: What are the units of angular velocity ω? Q: What are the units of angular momentum L?
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.