A trip to Buffalo in a solar-powered car -- the solution

Your friend Herbie lives in Buffalo, a distance d = 75 miles from your home in Rochester. On a nice Saturday, you decide to drive to his place for a visit.

But instead of the usual car, you decide to make the trip in a solar-powered racer!

Image of the "Tokai Challenger" courtesy of Wikipedia

When you hit the road at 6 AM, the Sun is barely up above the trees, and your car barely creeps forward. But as the Sun rises, the car goes faster. In fact, the speed rises in a very regular manner:



      6 AM       v  =   0 mph

      7 AM       v  =  10 mph

      8 AM       v  =  20 mph

      9 AM       v  =  30 mph

     10 AM       v  =  40 mph

... and so on. In fact, you can describe the speed of the car in the following manner, where t is the time in hours since your trip began.



  Q:  How long does it take to reach Buffalo at this constant acceleration?

Which of the 1-D kinematic equations is the best choice here? I think this one:

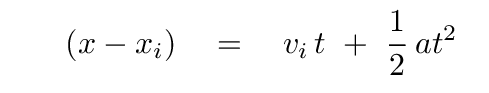
Let's replace the general terms in the equation with our variables: the distance d from Rochester to Buffalo is the displacement, and the initial velocity is zero, so we can write

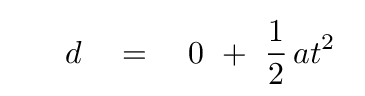
Now we use algebra to move the quantity for which we'll solve -- the time of the trip, t -- to the left-hand side; and compute!