Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
How many planets are there? The answer to that question depends on when you ask it. Let's look at how the answer has changed over time.
Q: If you asked an ancient Greek astronomer how many planets
there are, he would say ... ?
He would say 7, for the objects:
Q: If you asked Galileo how many planets there are,
he would say ... ?
Galileo would answer 6, listing
So the Sun and Moon have been removed from the list, but Earth has been added.
And there the list would sit for a few hundred years, until William Herschel recognized Uranus as a new planet in 1781.
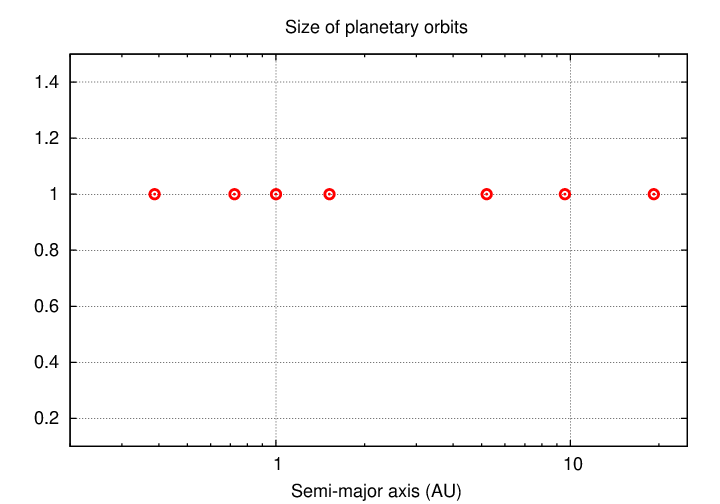
Now, astronomers at this time noticed that there was a bit of a "gap" in the distribution of the sizes of planetary orbits. It shows up most clearly if one plots the orbital sizes on a logarithmic scale:
Some scientists thought that there might be an additional object orbiting in the space between the orbits of Mars and Jupiter, and started searching for it systematically. One of these was Giuseppe Piazzi, who used a telescope with lenses just 7.5-cm (3 inches) in diameter -- not much larger than those in binoculars.
Image of the Palmero Circle taken from
Serio, Manara and Sicoli, in "Asteroids III", Arizona Press (2002)
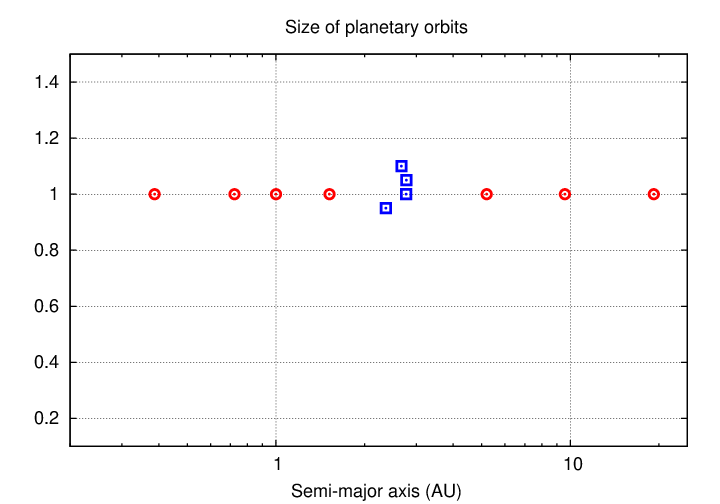
In 1801, Piazzi announced that he had found it. Other astronomers added their own measurements, and the orbit of the object turned out to have a semi-major axis of about 2.8 AU. Right in the gap! This must be the missing planet! Piazzi named this object Cere, after an Italian goddess.
So, now the Solar System had 8 planets:
But some scientists were puzzled. Ceres was much fainter than Mars or Jupiter, so it had to be much smaller. MUCH smaller. Sort of strange.
And then, within less than a year, things got stranger: another small object was found in 1802, orbiting the Sun at nearly the same distance as Ceres.
And then another in 1804.
And then ANOTHER in 1807.
Well, this didn't make any sense at all -- did it? Why should there be 4 tiny planets between Mars and Jupiter?
Nonetheless, the count of planets was now up to 11 by 1808.
By the mid-1800s, as astronomers continued to survey the skies and discover more of these small bodies, the count of planets had risen to over 20. One more big planet was found by this time, too: Neptune, predicted and then discovered in 1846. The number of planets was growing so large that the astronomical community couldn't take it any more.
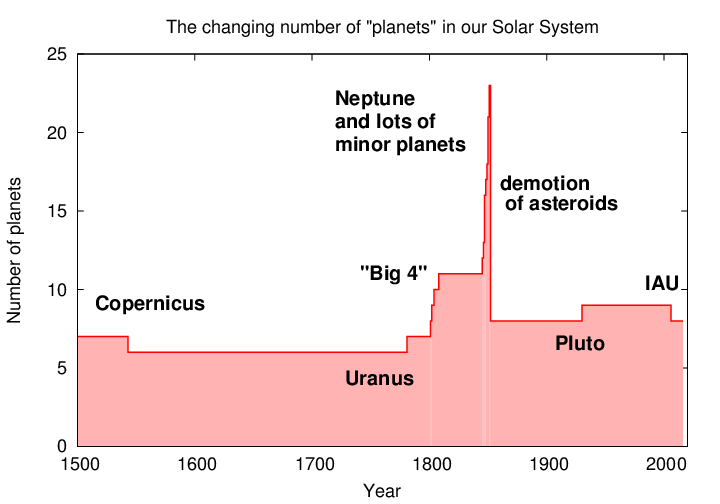
All the small bodies which had been discovered in that gap between Mars and Jupiter were now demoted: instead of planets, they would henceforth be called asteroids, which means "little stars."
That left the count of "real planets" at 8, where it would remain until 1930.
The simple answer would be "between the orbits of Mars and Jupiter." After all, that's where the first few hundred were found. But if we look at the full set of small bodies with orbits currently measured, we find a somewhat different answer.
Yes, a lot of the asteroids ARE found between Mars and Jupiter.
But you can see a small number of objects have semi-major axes which are less than 1.5 AU -- smaller than the orbit of Mars. And if we change the vertical axis to a logarithmic scale, to emphasize the smaller features, we can see that some asteroids actually have orbits smaller than that of the Earth.
Q: Is there any possible problem with asteroids
whose orbit is roughly the same size as that of the Earth?
Yes, there is a possible problem.
Image copyright
Mark Garlick
courtesy of
National Geographic Society
We'll return to this topic later.
But there are a few asteroids way out beyond the orbit of Jupiter, too.
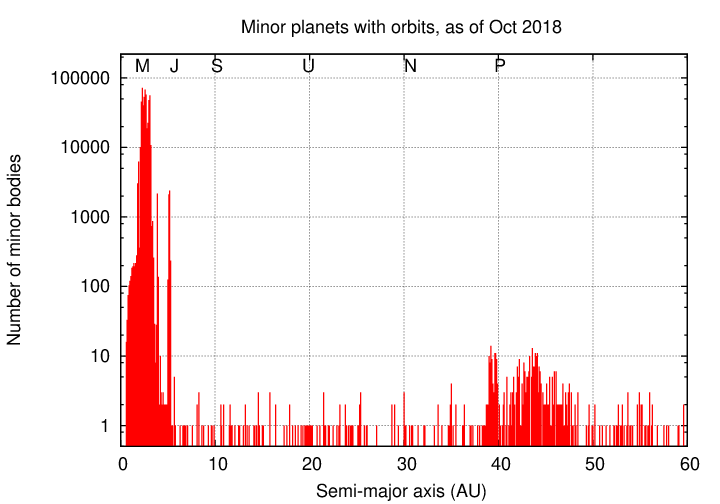
In fact, astronomers have found a very few out beyond the orbits of Neptune and Pluto, too.
In today's class, we will focus on the objects which are in the inner Solar System, no farther away than Saturn. We'll deal with the most distant objects -- which have different compositions and histories -- in several weeks.
Way back in the 1860s, when the number of known asteroids was still relatively small, Daniel Kirkwood noticed that they were not distributed evenly in the space between Mars and Jupiter. There appeared to be "gaps" in the number of asteroids at certain distances from the Sun.
It's pretty easy to see those gaps with the very large number of asteroids in today's catalogs:
But why are there gaps? What's wrong with those particular orbits?
The key issue is that certain orbits are resonant with the orbit of Jupiter. What does that mean? It means that certain orbits will cause an asteroid to return to the same location in its orbit just as Jupiter happens to be in the same relative position.
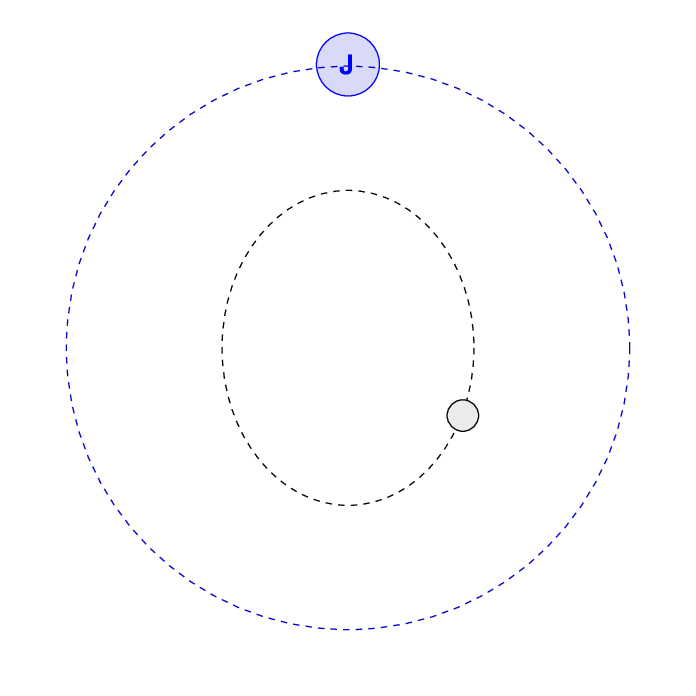
Consider an asteroid whose period is exactly HALF that of Jupiter. Suppose the asteroid has slightly ellpitical orbit.
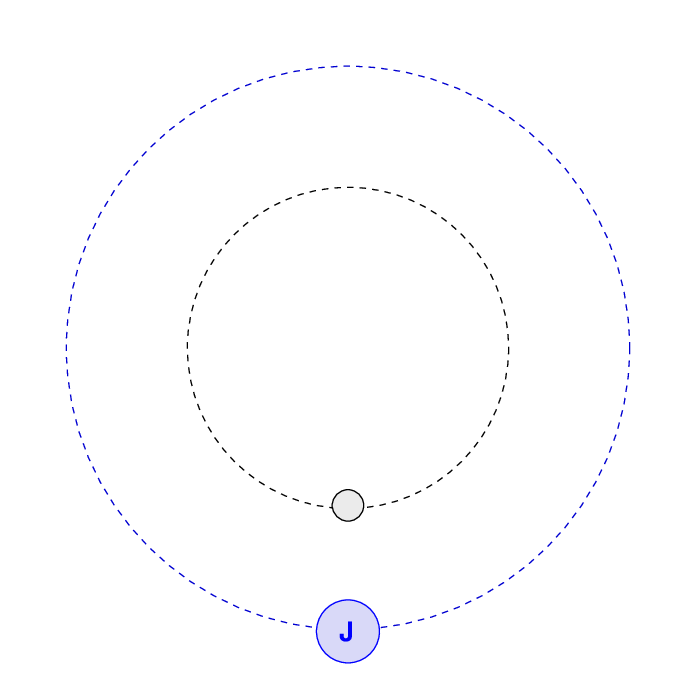
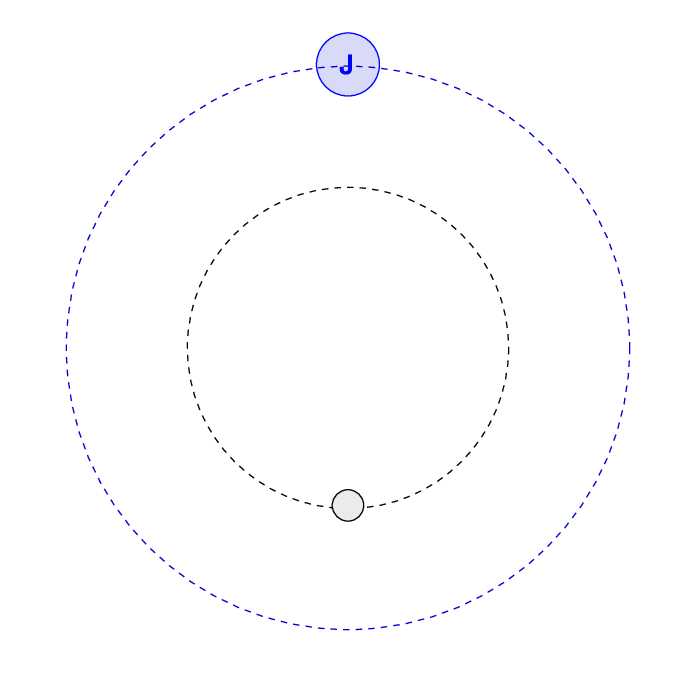
In this arrangement, every time the asteroid returns to one of the extreme points in its orbit, Jupiter is sitting right next to it. That means that Jupiter's strongest gravitational pull, when the two planets are closest to each other, always occurs at this same point in the asteroid's orbit.
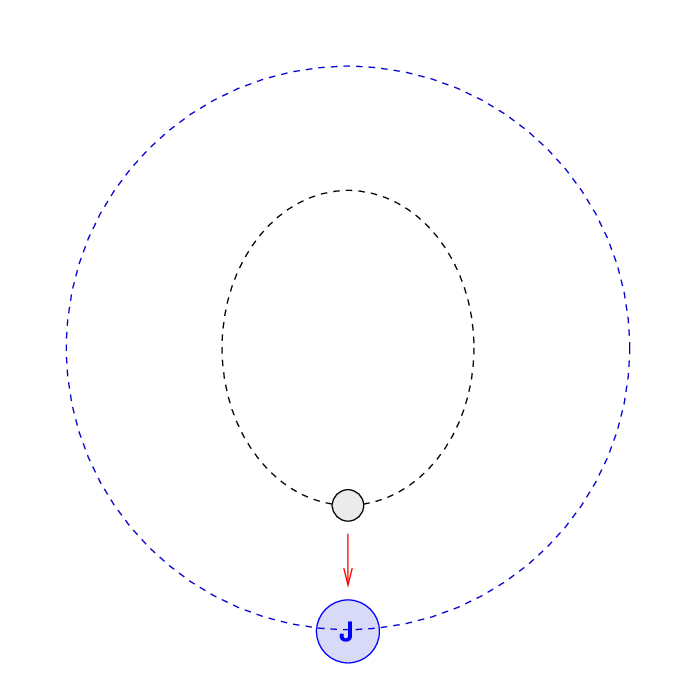
What will that strong gravitational pull do? If it always occurs when the asteroid is in this part of its orbit, then the force will nudge the asteroid to a slightly larger, more elliptical orbit.
And so, the size of the asteroid's orbit will increase. If these perturbations cause any asteroid with a similar orbital radius (and orbital period) to move to a different orbit, then we end up with a "gap" at that orbital radius.
On the other hand, if an asteroid has an orbit with a different period -- one that is NOT a half (or a third, or a quarter, or other simple ratio) of Jupiter's period -- then what will happen to it?
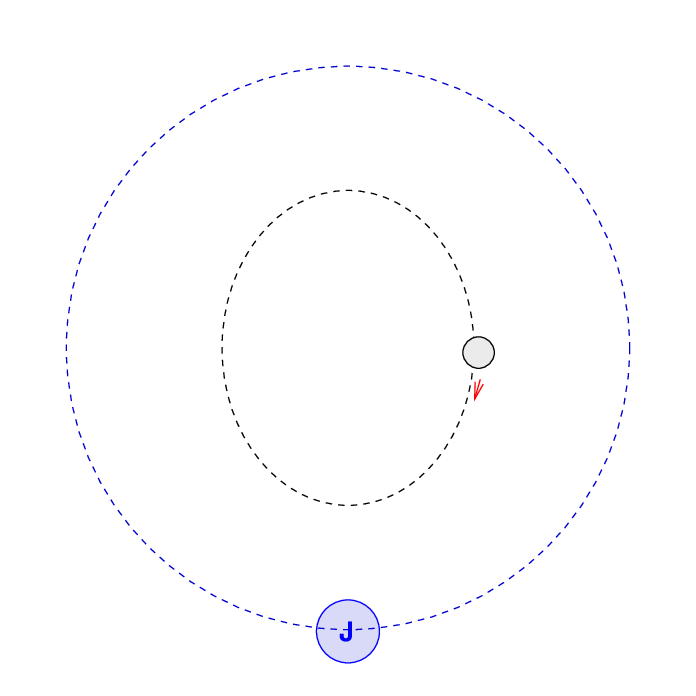
Let's find out. I'll create an asteroid whose orbital period is a little bit MORE than half of Jupiter's.
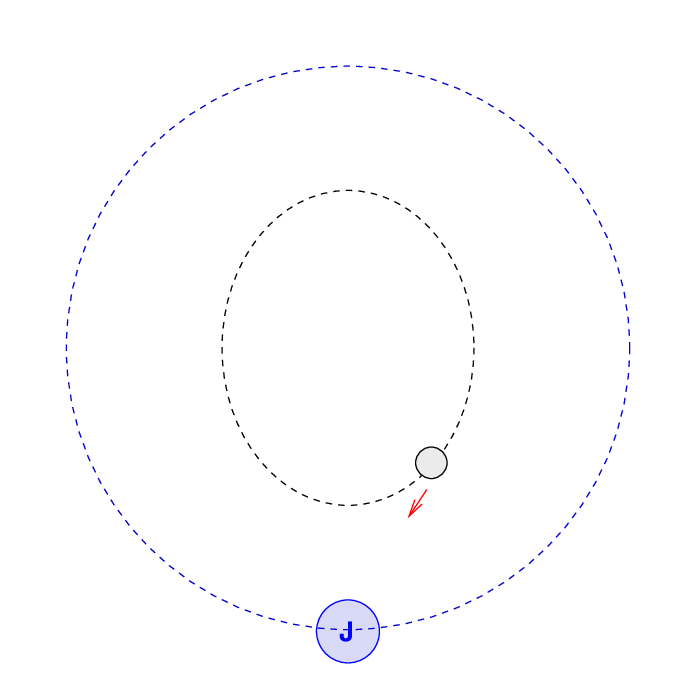
After one full period of Jupiter, the asteroid has not yet caught up to Jupiter again. So, the gravitational nudge from Jupiter does not come when the asteroid is at its maximum distance from the Sun.
And if we wait another orbital period of Jupiter, the asteroid is even farther away from the extreme of its elliptical orbit.
The bottom line is that orbital perturbations will build up and modify the orbit of an asteroid only if the asteroid's orbital period is a simple ratio of Jupiter's orbital period.
For example, suppose that the asteroid's orbital period is exactly HALF Jupiter's period.
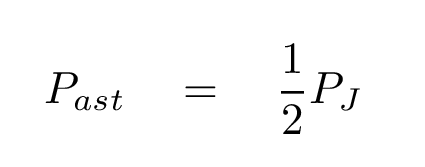
What orbital radius would cause that to happen?
Q: Is it possible to figure out how the semi-major axis of
this asteroid's orbit is related to that of Jupiter?
Sure! Just use good old Kepler's Third Law.
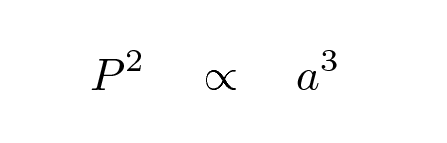
We can do a little algebra:
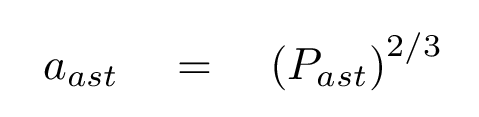
So, if I know the orbital period of Jupiter -- which is PJ = 11.9 years -- then I can figure out the orbital radius of an asteroid which has half of its period:
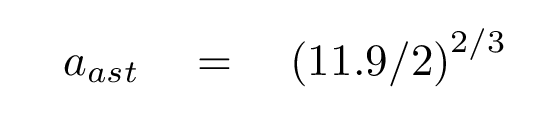
And that works out to a = 3.284 AU.
Do you see any gap in the distribution of asteroid semi-major axes at this value?
Okay, it's your turn:
Q: Try using some other simple ratio of periods of asteroid to
Jupiter, and follow the same method to find the
semi-major axis which yields that ratio.
Can you explain the other gaps?
One of those gaps is rather tricky ...
Not all asteroids are identical; in fact, not all of them are "rocks." How do we know? Well, we have three sources of information:
This iron meteorite was actually found on the surface of Mars!
Image courtesy of
NASA/JPL
Image courtesy of
H. Raab and Wikimedia
These may look like ordinary stony meteorites on the outside (like this one, being collected in Antarctica),
Image courtesy of
French, MacPherson and Clarke (1990), and
Righter (2010)
but if one slices them, one will find a rich set of little chunks, or "chondrites", of material.
Image courtesy of
French, MacPherson and Clarke (1990), and
Righter (2010)
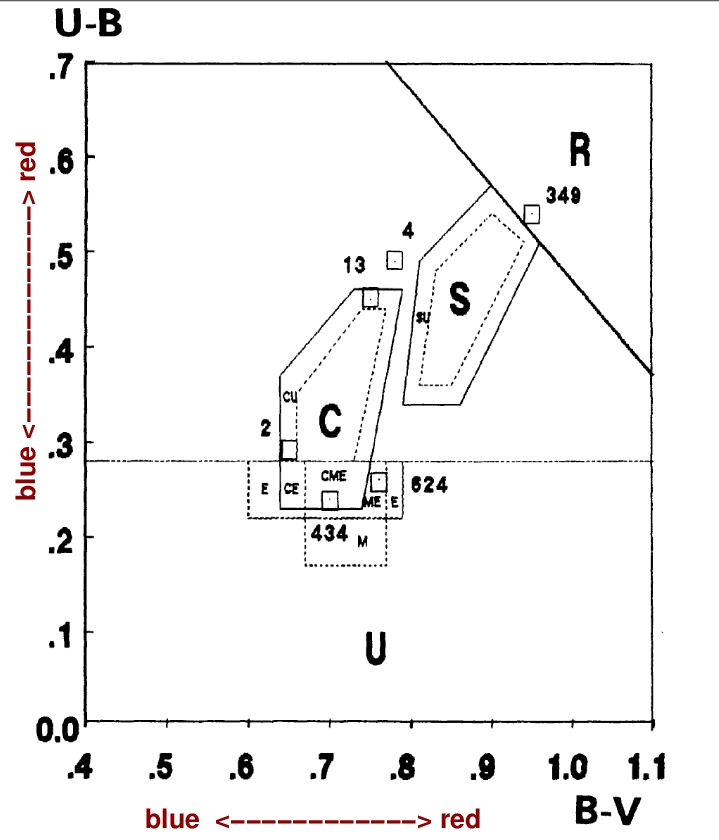
Figure 1 taken and modified from
Hollis, JBAA 104, 112 (1994)
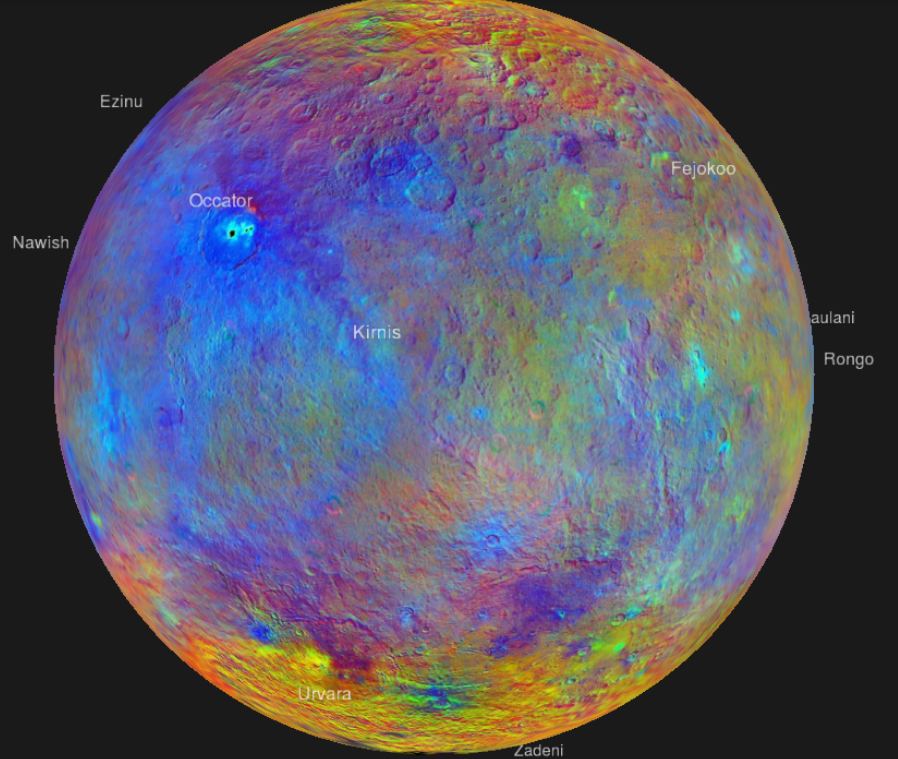
Image of Ceres courtesy of
NY Times and NASA/JPL
Map of Ceres courtesy of
NASA/JPL-Caltech/UCLA/MPS/DLR/IDA
When we put all the information from these various sources together, we can reach several conclusions:
Yes, some asteroids, like Ceres, are now (or were long ago) large enough to form a body with chemically different layers. But were they large enough to form a real, Earth-sized planet?
Q: If one stuck together all the asteroids, would they create
a solid body as large as the Earth?
Is it possible that there once was a planet between Mars
and Jupiter, which exploded to form the asteroids?
Well, look at how large the two biggest asteroids are compared to Mars.
Estimates of the total mass of all the asteroids added together come to less than 1022 kg. That's less than one percent the mass of the Earth.
So, no. No extra planet exploded to form the asteroids. Unlike Alderaan.
Image from
Star Wars, of course.
Yes, asteroids can be a danger to life on Earth. Just ask this guy:
Image courtesy of
Allie_Caulfield and Wikimedia
Oh, wait, you can't ask him, because he is DEAD.
Asteroids are basically rocks and pieces of metal. We don't normally worry about rocks. What makes asteroids more dangerous than typical rocks? Well, two things:
The kinetic energy of an object depends both on its mass (that's the BIG part) and on its velocity (that's the FAST part), in the following way:
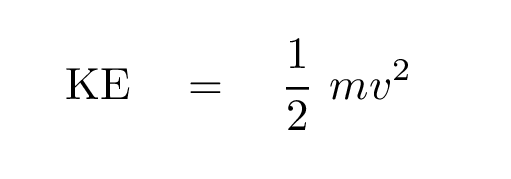
Let's try using this formula to compute the kinetic energy of, say, a lacrosse ball. It has a mass of about 0.15 kg.
speed (m/s) KE (Joules)
-------------------------------------------
10
100
1,000
10,000
100,000
-------------------------------------------
Some of those numbers look pretty big. But what do they mean? Just how much energy is one Joule, anyway?
It turns out that 1 Joule is a pretty small amount of energy. I wouldn't not be scared of a rock with 1 measly Joule. But objects with millions, or billions, of Joules -- those can be dangerous.
For example, you probably know better than to fool around with the explosive known as TNT, right?
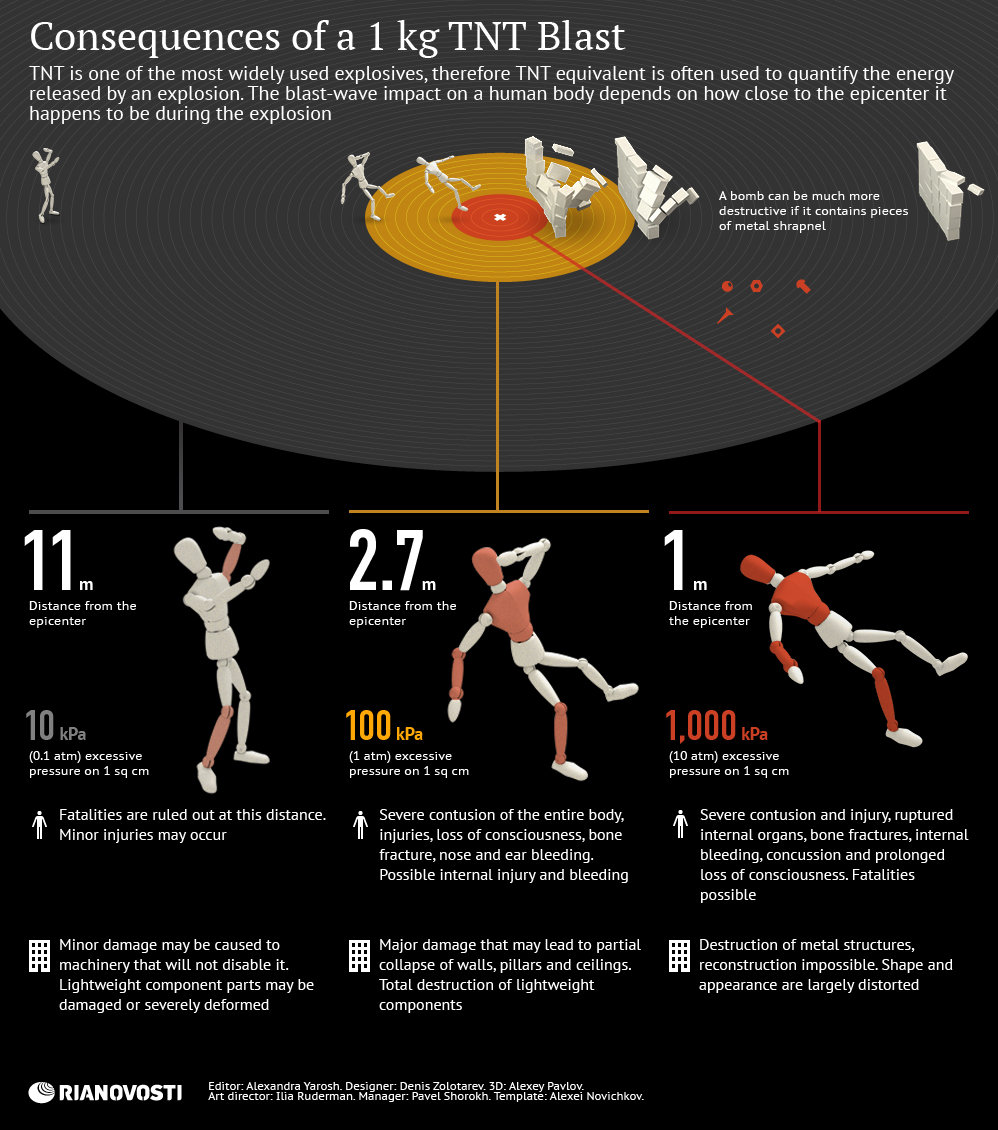
Image courtesy of
Sputniknews.com
Well, if you were to gather ONE TON OF TNT and blow it all up at once, you would release a kiloton of energy = 4.2 x 109 Joules.
Q: How fast would a lacrosse ball have to move in order
to have a kinetic energy of 1 kiloton?
The good news is -- that's faster than any asteroids move in our Solar System. Typical asteroids will encounter the Earth at relative speeds of "only" 10 to 35 km/s.
The bad news is -- many asteroids are larger than a lacrosse ball. Much larger. The object known as the Chelyabinsk Meteor was probably about 20 meters in diameter, with a mass of 12 million kg, moving at about 19 km/sec.
Q: What was the kinetic energy of the Chelyabinsk Meteor?
Image taken from
Youtube: Meteor Hits Russia Feb 15, 2013 - Event Archive
Back in 1908, a considerably larger object -- maybe 60 to 200 meters in diameter --- struck the remote Russian steppe near Tunguska. The results were more than a light show. Trees were knocked down over a region of hundreds of square kilometers.
Image courtesy of
GEO magazine
Astronomers have been scanning the skys systematically in the past few decades to look for asteroids with orbits that might bring them close to Earth over the next few hundred years. So far, we haven't found any ... but we are still working on it.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.