 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
There are several different techniques for acquiring spectra of exoplanets and their atmospheres. In brief, they are
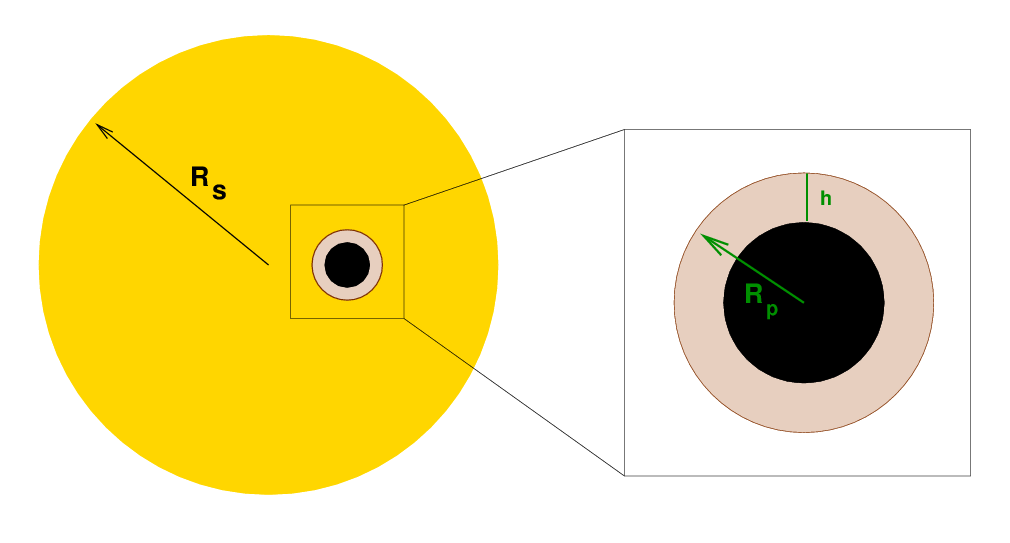
This is the really interesting option, in my opinion. As a planet passes in front of its star, oodles of light from the star fly through the atmosphere, probing it thoroughly at a range of depths.
One of the problems is that only a tiny fraction of the star's light goes through the planet's atmosphere.

Q: Write an equation which expresses the fraction of
observed light transmitted by the
planet's atmosphere.
Q: For a typical Hot Jupiter, what is this fraction?
Q: For an Earth-sized planet, what is this fraction?
The expected signal is very small (as is always the case with exoplanet science). That means that one must perform very careful calibration and data reduction. Fortunately, since a transit only lasts a few hours, it will often be possible to acquire the in-transit vs. out-of-transit comparison data during a single session on a single night.
Let's look at a Good Case: Spectrally resolved detection of sodium in the atmosphere of HD189733b with the HARPS spectrograph by Wyttenbach et al. (2015). Fig 1 from their paper shows the importance of removing features caused by the Earth's atmosphere. On their "Night 3", they had to perform two rounds of corrections because the properties of the air had changed from one night to the next.
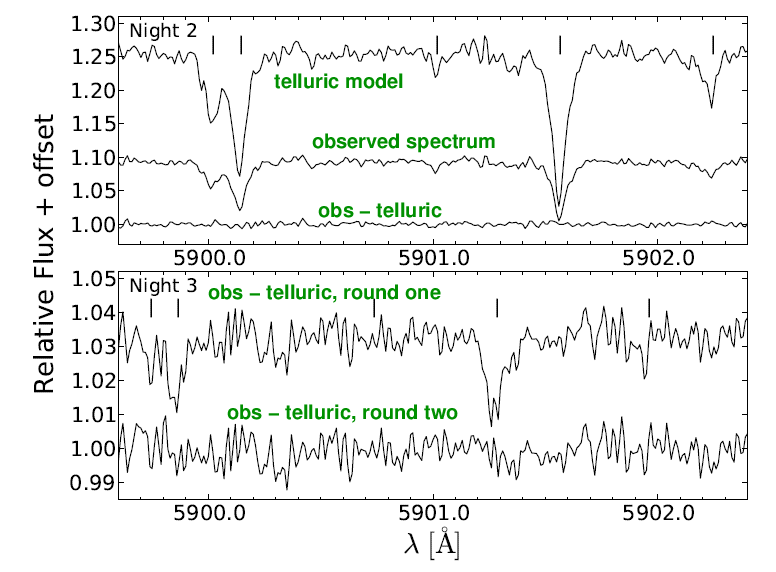
Fig 1 modified from
Wyttenbach et al., arXiv 1503.05581v1
However, the payoff is great: after performing all the calibrations, and co-adding spectra taken over three nights (each night including some inside and outside of the transit), the authors find a clear detection of sodium absorption lines due to the planet's atmosphere:

Fig 2 modified from
Wyttenbach et al., arXiv 1503.05581v1
Note the change in the vertical scale from the top panel to the bottom panel!
This group used the high-resolution HARPS spectrograph on the ESO 3.6-meter telescope at La Silla, Chile. Compare their results to earlier measurements (Huitson et al., MNRAS 422, 2477 (2012) made with the 2.4-meter HST and STIS spectrograph. Big, special-purpose, high-resolution spectrographs can yield excellent results.
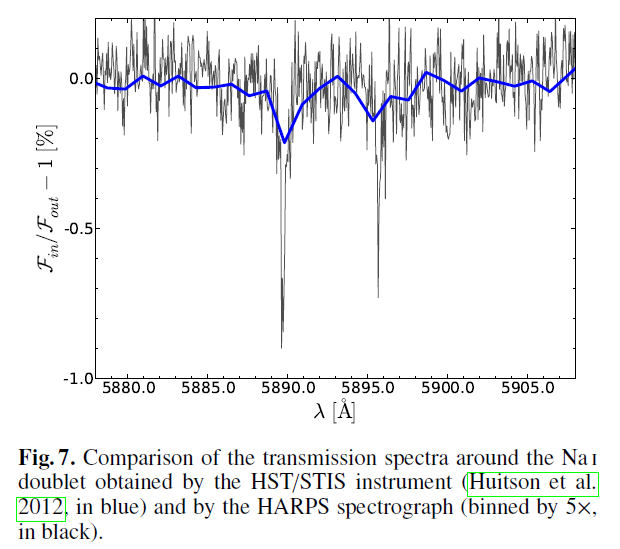
Fig 7 from
Wyttenbach et al., arXiv 1503.05581v1
Let's take a moment to review the observations. This star, HD 189733, is roughly V = 7.6. The authors spent three nights observing the star -- measuring one transit each night -- and then combined their results. They DID detect a feature due to the planet's atmosphere, clearly. Do the numbers add up?
Recall that a V = 0 star produces about 10^6 photons
per square cm. per second in V-band.
The authors used a telescope with radius 180 cm.
Q: How many photons should they have collected per second?
Q: In order to see a feature with fractional depth approx = 10^(-4),
how many photons must they collect?
Q: How long should they need to expose?
Q: How long _did_ they expose?
Q: Why the difference? Have we forgotten something?
But the previous example was one of the best detections of an atmospheric feature I've seen. More typical, perhaps, is the following, taken from Sedaghati et al., A&A, 576, L11 (2015) . They used the FORS spectrograph on the 8-m VLT to observe the planet WASP-19b as it moved in front of its star.
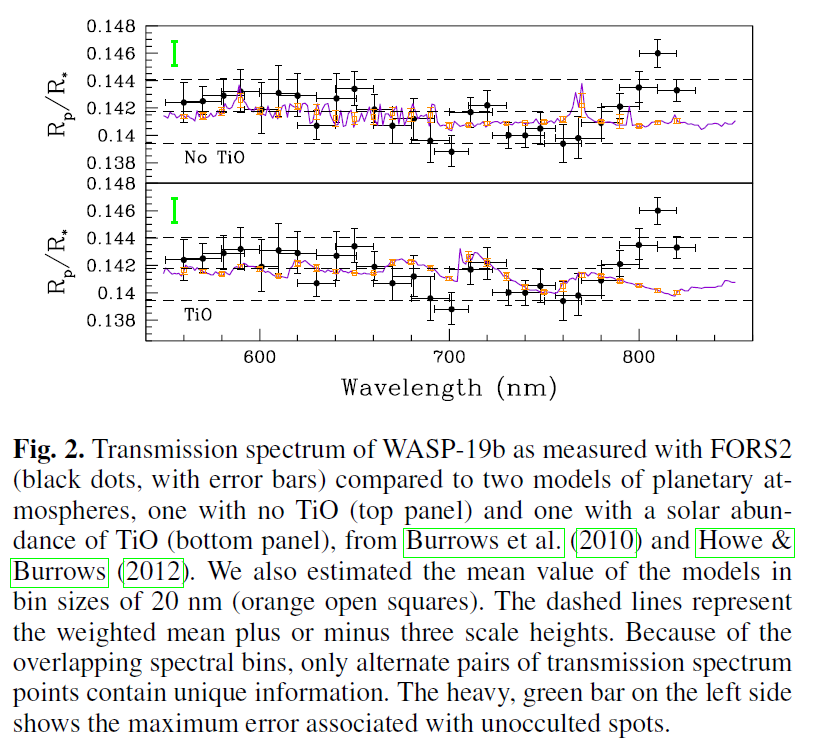
Fig 2 from
Sedaghati et al., A&A, 576, L11 (2015) .
Q: Do you conclude that this planet has TiO in its atmosphere?
And in some cases, it turns out that we seem to move BACKWARDS the harder we try. Consider the case of Hoeijmakers et al., A&A 575, 20 (2015). They tried to measure absorption by TiO in the atmosphere of HD 209458b during a transit, using the 8.2-m Subaru telescope and its High Dispersion Spectrograph between 5500 and 6700 Angstroms.
First, they found no positive result when they compared their spectrum of the planet's atmosphere to a model spectrum with TiO.
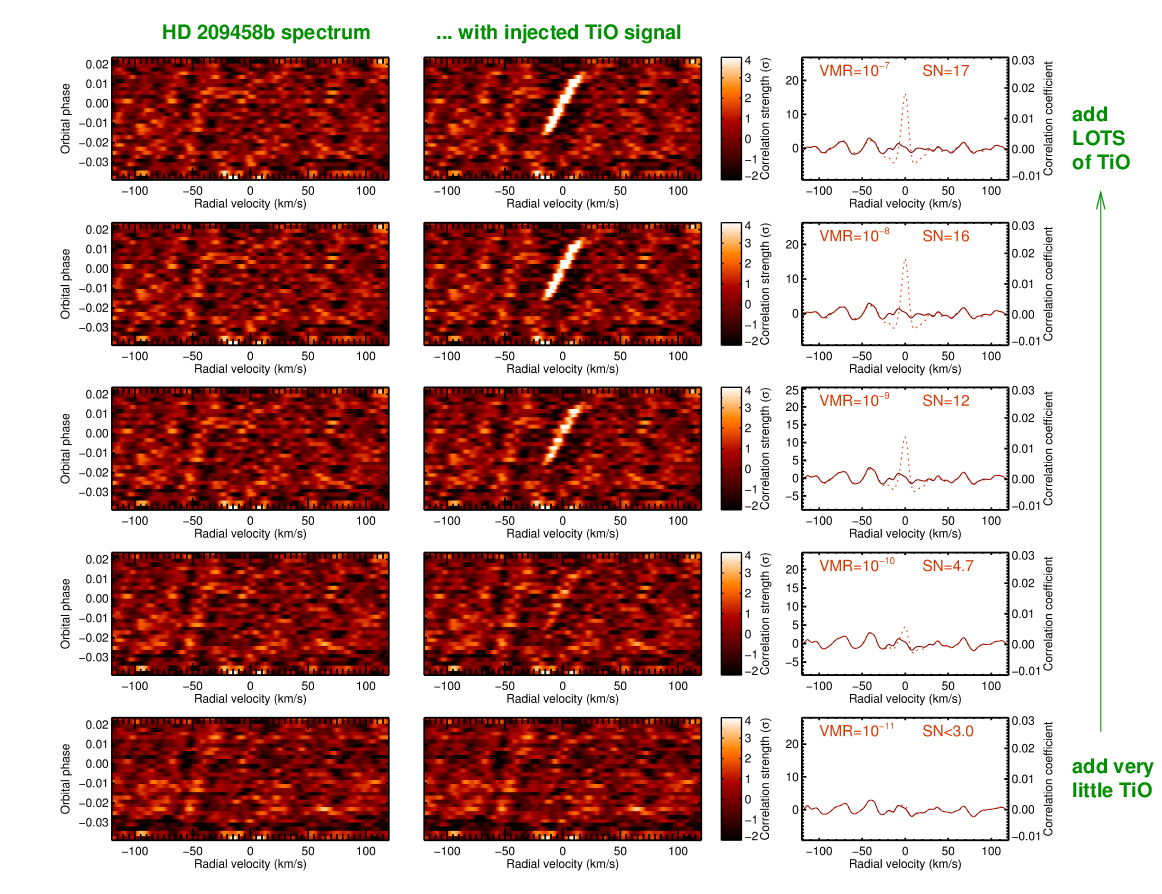
Figure 5 modified from
Hoeijmakers et al., A&A 575, 20 (2015).
But the situation is worse -- when they used the same equipment to observe Barnard's Star, which is KNOWN to have plenty of TiO in its very cool stellar photosphere, they could not reliably detect the molecular features!
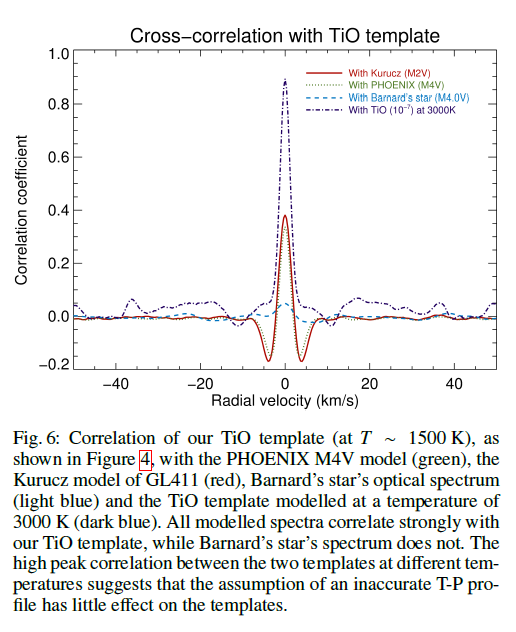
Figure 6 modified from
Hoeijmakers et al., A&A 575, 20 (2015).
The authors tracked the problem down to an issue with the line lists for TiO. Apparently, the measured (or calculated?) wavelengths of the many, many, many transitions of this molecule may contain a number of inaccuracies, enough to confuse the correlation procedures.
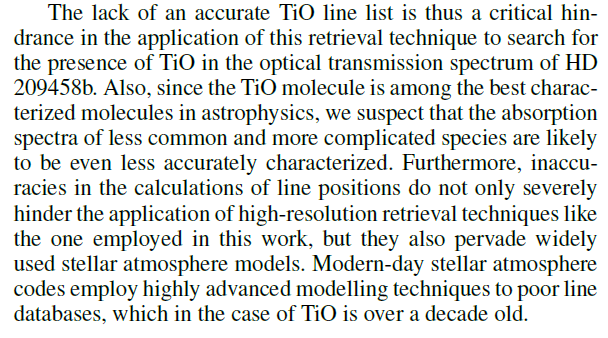
Text taken from
Hoeijmakers et al., A&A 575, 20 (2015).
As we try to study exoplanetary atmospheres in more detail, we learn that we need to learn more.
The basic idea here is to measure the light reflected by a planet from its host star. Of course, in almost every case, this planetary contribution will be mixed with the direct light of the star itself, because the host and planet are separated by only tiny tiny fractions of an arcsecond. Therefore, we face the problem of contrast: trying to identify the tiny fraction of the combined light which is due to the planet alone.
Just how much light might we expect to see for a typical situation? Consider a hot Jupiter around a Sun-like star.
Radius of star: 7 x 108 m
Radius of planet: 7 x 107 m
Orbital radius: 0.05 AU ~ 7 x 109 m
Albedo: 0.3
Q: What fraction of the star's light strikes the planet?
Q: What fraction of the star's light can be reflected to the observer?
Yes, that's a very small fraction, isn't it? Trying to detect planets via the light they reflect is a difficult task.
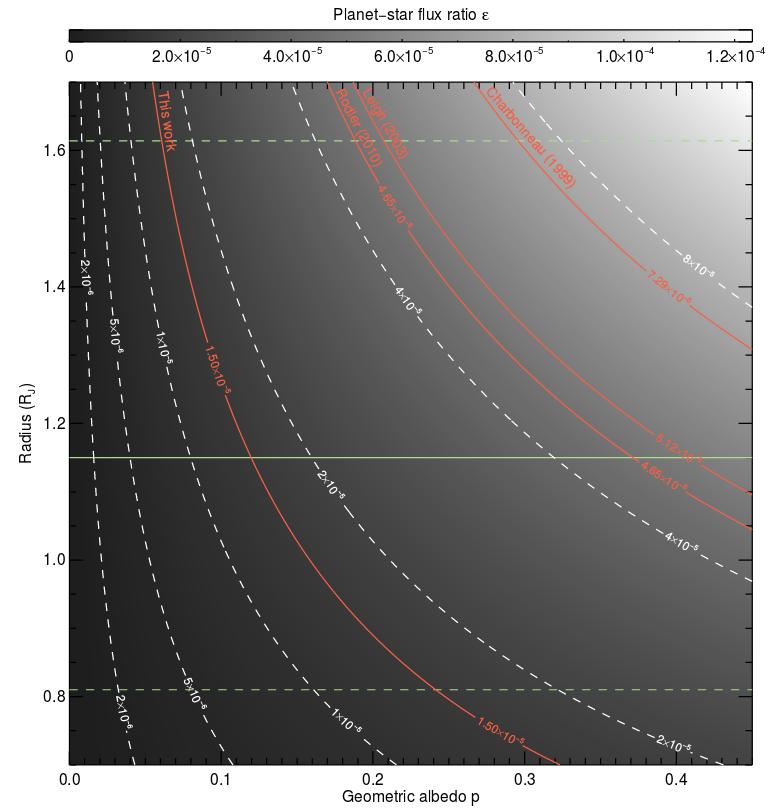
Figure 13 taken from
Hoeijmakers et al., arXiv 1711.05334 (2017).
One of the most comprehensive attempts to do so is described by Hoeijmakers et al., arXiv 1711.05334 (2017). This team combined the spectra taken by a number of different observatories, over a period of several years. They used the known properties of this planet's orbit to compute the radial velocity of the planet at the time of each spectrum; Note that this Doppler shift is a key to separating the light from the planet and the light from the host star. If you know the planet's motion, then you might able to recognize its (weak) signal, even in the presence of noise from the much brighter host.

Figure 7 taken from
Hoeijmakers et al., arXiv 1711.05334 (2017).
So, the group collected all the spectra from different sources, shifted each spectrum to remove the Doppler shift, then co-added all the aligned spectra. They then compared this co-added spectrum to several model spectra; even if individual spectral lines might be too weak for the eye to detect, the cross-correlation of the co-added spectrum with a model spectrum (placed at the proper velocity) ought to show a peak due to the combination of many lines.
Do you see the expected peak?
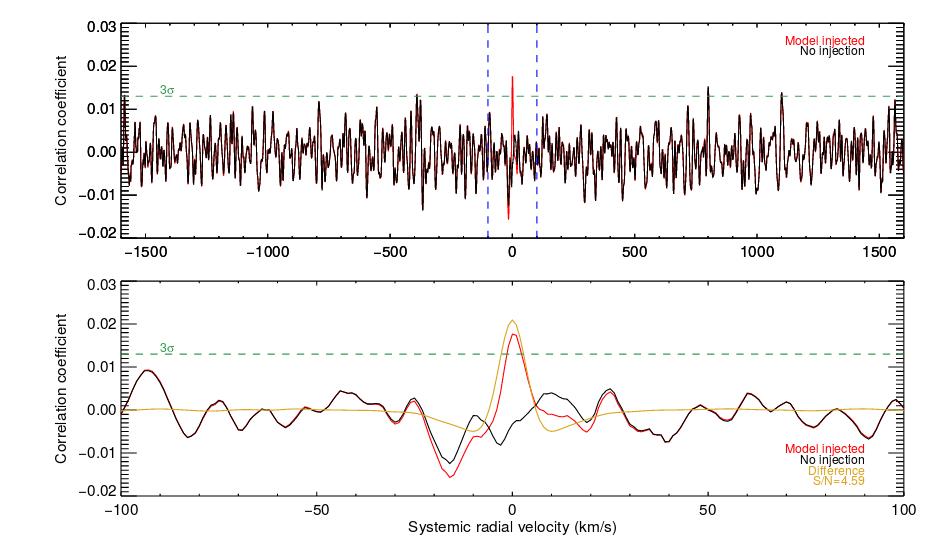
Figure 9 taken from
Hoeijmakers et al., arXiv 1711.05334 (2017).
Consider a planet orbiting close to its star. If the planet is hot, either from its own internal heat sources or due to the stellar radiation, it will emit light as a blackbody. How bright will it appear compared to the star?
Well, if we treat both the star and planet as blackbodies, the ratio of their fluxes will be
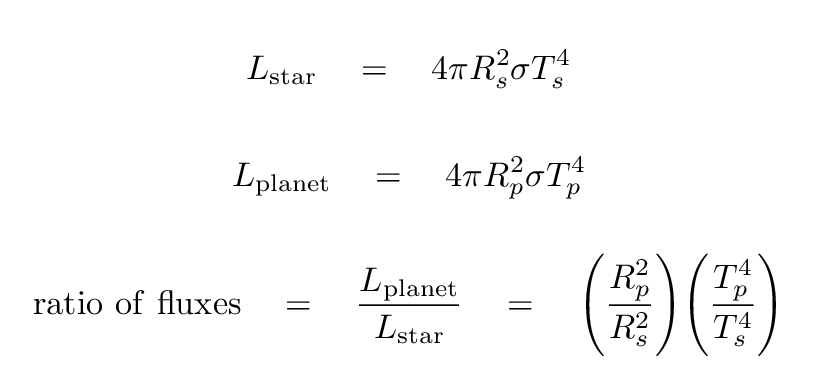
Q: Compute this ratio for a typical Hot Jupiter
around a sun-like star.
Q: Compute this ratio for an Earth-like planet
in an Earth-like orbit
around a sun-like star.
It's clear, once again, that detecting even the biggest, brightest planets will be no easy matter; and that detecting Earth-like planets is, in the words of Brother Maynard, Right Out!
Still, let's look at an example of this technique in action. You'll see that to call this "spectroscopy" is a bit of a stretch; it might be better to call this thermal extremely low-resolution spectroscopy.
Our example is a study of the planet around HAT-2-P by Lewis et al. They compare Spitzer measurements of this planet in the near-IR to a number of atmospheric models. The planet is big (8.9 times the mass and 1.1 times the radius of Jupiter) and close (period = 5.6 days, semi-major axis = 0.07 AU) to its host star; in other words, a pretty standard Hot Jupiter.
But note that this system is NOT as simple as we often pretend. In particular, the orbit of the planet is not circular.
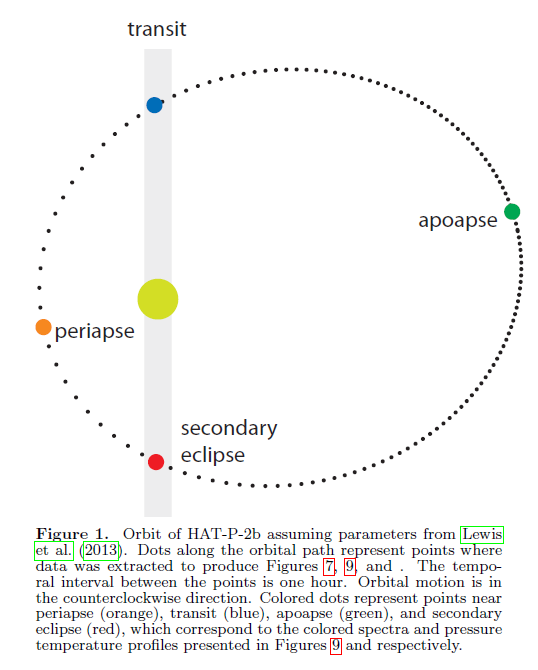
Fig 1 taken from
Lewis et al., ApJ 795, 150 (2014)
This change in distance from the host star means a big change in the effective temperature of the planet over the course of its orbit. Remember that the orbital period is just 5.6 days.
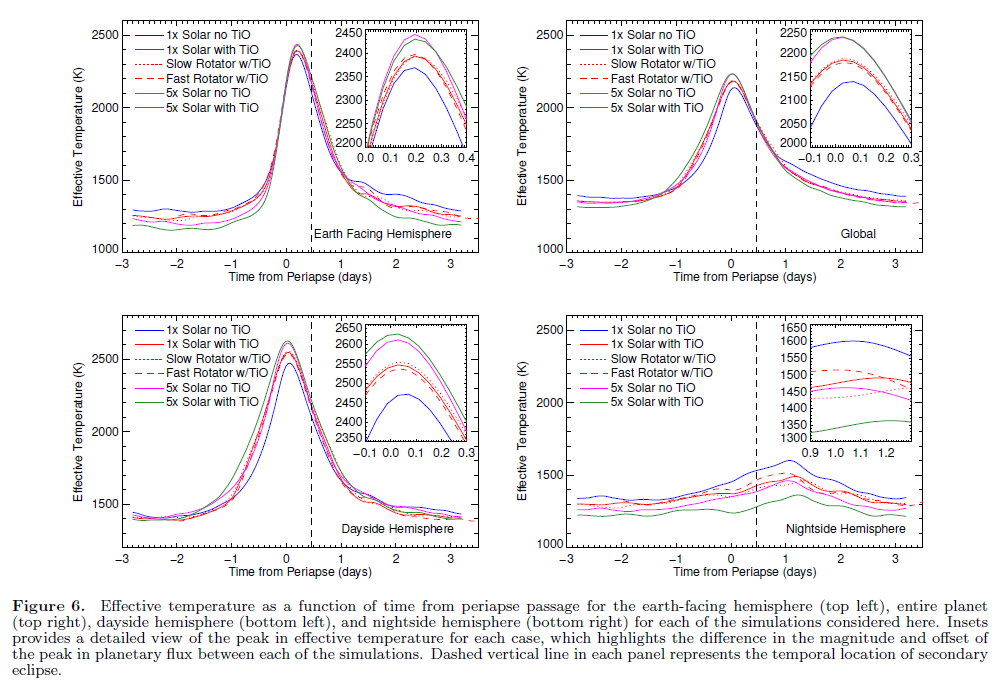
Fig 6 taken from
Lewis et al., ApJ 795, 150 (2014)
So, what do the authors actually measure with Spitzer? Their measurements are shown in the figures below as the black symbols; all the other colored symbols represent the predictions of various models.
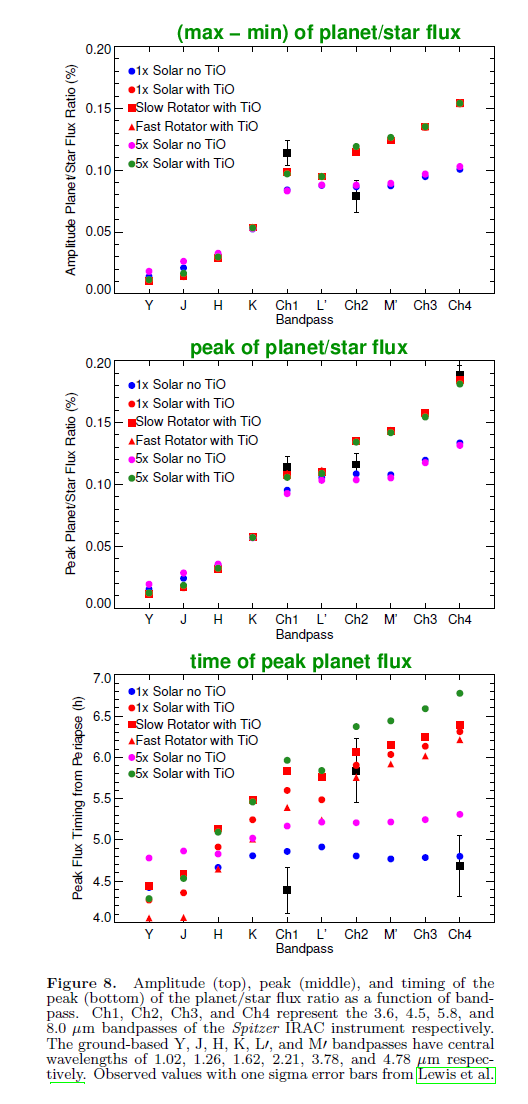
Among the conclusions of this study are:
Q: How many existing instruments can observe this system
at wavelengths longer than 4.5 microns?
 Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.
Copyright © Michael Richmond.
This work is licensed under a Creative Commons License.